md65536

Oops, I was thinking of gravitational redshift and often mistakenly call it gravitational Doppler shift. I was assuming that if there was a difference in the rate of time due to inflation, it would be due to a change in spacetime curvature. Can there be spatial flatness, but curved time, with a constant speed of light? Or would a changing rate of time require a changing speed of light? I think it does. I assumed "speed of time" meant rate, and interpreted it to mean "How would the rate of a clock now compare to the rate of the same clock shortly after the big bang (assuming the most general case possible)?" The more I think about it the less sure I am that it even makes sense to ask. For example it makes sense to say, "I've aged one year less than my twin because of those two years (Earth time) I spent traveling when I aged at half the rate that I'm aging now," but this only makes sense with that second clock to compare to. In another frame, I'm aging at a half rate now, relative to some time I was traveling. Trying to "define things in a vacuum" as you mentioned, I was always aging 1 yr/yr and never aged slower. But then again!, I can just treat myself now and in the past as 2 different clocks and I can compare them, basically label my past self my "twin", and it's fine to say my past self in a different reference frame aged slower relative to me now, and for my past self to say "my future self will age slower relative to me now!" But back to the case of inflation, I was thinking maybe it's possible to measure your own past clock relative to your current clock. For example if you could define a light clock where the only thing that changes over time is gravitational time dilation (is that even possible to do?), then there might be a red or blue shift occurring in the light clock over time. But how? For example you could make the clock inertial, and say it has 2 mirrors and you keep the mirrors relatively stationary throughout time. But if space is expanding during that time, keeping them stationary for one observer (you) means moving the mirrors according to another observer, so are you really making gravitational time dilation the only thing that affects the redshift? Anyway I got stuck without figuring that out. Without a way to measure it, I can't make sense of what it means to compare the rate of a clock in the past with itself now. Using another clock to compare to is fine, the "standard clock" you asked for, but like you say: how? For example in flat spacetime in SR you can define a reference clock free from the effects of time dilation simply by making it inertial. But how would you bring a reference clock through an era of inflation without having it affected by it?

Gravitational potential is only meaningful in some simpler metrics like Schwarzschild. Assuming our universe is flat on the largest scales, do we say it's flat because it has uniform mass distribution, and is that independent of the total mass? Then, assuming it's flat and that the big bang happened, theoretically it was always flat? Or is it flat specifically because it has very little average density, and wasn't always flat? If what I wrote above makes sense, you'd be trying to compare a clock in flat spacetime, to a clock in flat spacetime after inflation. Could it make sense to do it with a Doppler analysis? If you compared clocks at different places, the Doppler shift would mostly be due to inflation, but would there also be a gravitational shift over time even for a single inertial clock? If gravitational time dilation requires curvature, and the curvature of the universe didn't change in general, then I would guess there's no measurable change in the rate of time for an inertial clock (in the general case) now relative to the same clock earlier, and no theoretical change either.

The transformation doesn't tell you what x and t are, you can choose that and it works for anything. If you make t a constant, then x(t) can describe a single event. You don't need objects at all. x = vt+r for a constant r gives you the world line of an object that is at rest relative to O' (the x coordinate changes over time, but x' doesn't). x = r gives you the world line of an object that is at rest relative to O (the x' coordinate changes over time). x = 2vt is an object not at rest relative to either. x can be independent of v. So: no, there's no implicit object that is moving at v, it is the reference frame O' that is moving at v.

With x and t being anything, the case where x=vt describes a particle that is at the origin of O' at time t, and the meaning of gamma(x - vt) = 0 is that a distance of 0 will always be 0 no matter what the length contraction factor, or in other words if two things are at the same place and time, you can't change that just by transforming their coordinates. Conversely if you have 2 events 1 m apart, you can transform to another coordinate system where they're 0.5 m apart. Eg. let x=vt+1 be a particle that is 1 unit (according to O) away from the origin of O', then x' will depend on gamma.

In general and specifically in our universe, a frame of reference in curved spacetime is a strictly local thing, right? There's no global frame of reference that can be used to consider all the energy in the universe. The best we can do is say that for a local frame of reference, what we measure or predict is either consistent or not with the speculation that the total energy of the universe is zero. As far as I know, everything is consistent with it being 0, but there are too many unknowns that we can't measure, to say that it is so. You can talk about the total energy of the universe, but not in terms of a frame of reference. So if you have a model where say the universe spontaneously comes into existence from nothing, and energy is conserved, and it ends up with curved spacetime and no global frames of reference, there are still ways to describe the energy of that system being 0, but it wouldn't be described using things like a conservation law that applies to frames of reference. There are different descriptions of energy, some frame dependent and some invariant. Is this right?

You could choose a frame of reference where the entire universe has some great momentum, just by using an observer that's travelling at high speed relative to most stuff. It probably doesn't mean much to give a net momentum to the entire universe (because that's relative to what?). Or you could choose a frame where the universe has no net momentum. If you can do that, you can consider the "invariant energy" of the universe. Adding to what joigus wrote, I think anywhere you are in the universe you can define an observer where, measured locally, the net momentum of the universe is zero? I hope this isn't a gross misrepresentation of GR. Basically, such observers everywhere don't share a frame of reference with each other, because space is expanding between them, but they're also not moving through space relative to each other, so they can each be "at rest relative to the local zero-momentum frame of the universe"... I think.

Well it depends on what you're looking for, a model that shows things in a simple way, or a rendering of what would be seen by your eyes. The movie Interstellar shows visual effects around a black hole, and I think they needed a lot of time on a supercomputer to render them. In the SR game above, it doesn't include all the effects of SR, and yet the result is still confusing enough to the eye that you can't easily see what's going on. For Interstellar, they ignored the Doppler effect for artistic reasons. So even these are simplifications relative to what would actually be seen.

Edit: I missed the double-negative and only now see I'm agreeing with you, but I can't find where/if the statement you're quoting was resolved in this thread... How can you one possibly think that it follows, when you can do experiments in the kitchen sink with water and glasses that show that it's complete nonsense? You're Others are forgetting (but it looks like mistermack pointed it out long ago) that the space displaced by the mass that you're floating, does not need to be filled with mercury! So for example, say you have 700 kg of mercury in a container, filled right to the top, and you put in a 600 kg weight and it floats, displacing 600 kg of mercury. This is acceptable, agreed? However, the 600 kg of mercury has spilled over the top, so now you have a 600 kg weight floating on 100 kg of mercury, in the space that can hold 700 kg of mercury. Having tight seals or an "already floating" mass doesn't matter. If you have a stone sitting in a container that is just a little larger than the stone, you can float it using as little mercury as it takes to fill up the space not taken up by the stone.

This demonstrates visual effects of SR by the way, not GR, and is not applicable to this thread. I wasn't paying attention to which topic I was in!

There's http://gamelab.mit.edu/games/a-slower-speed-of-light/ It doesn't have a lot of options but it's built on an open-source Unity toolkit. The description says it shows time dilation (objects should be slower the closer you are to the speed of light) but I don't see it, and I also don't see delay of light (objects should appear faster as you approach them); it might be the effect is too small for me to notice.

Based on context and the forum we're in, the model must be a relativistic one. The quantum argument sounds reasonable but you can't make that claim about a gravitational singularity without a theory of quantum gravity. I don't see how lack of a clear cause is a useful argument. If you use that reasoning, you can claim that spontaneous particle decay can't happen at all. Yes, it seems accepted theory is moving away from the idea of an initial singularity. My limited understanding is that the singularity is considered speculative, shown to be not necessary in some theories, and not possible in others. However the issue isn't settled because to do so would require a theory of quantum gravity. Therefore making claims as if it's settled, is speculation only. I haven't followed OP's arguments, I'm only addressing yours.

Why not? Are you implying that a point particle can't decay?

I think this is the key to understanding it. I also think most people's approach is not best, whereby they imagine a contraption and explain if or how it works. If instead you ask yourself, given that the air is still and the ground is moving at a constant rate relative to it, is it possible to extract usable energy from that? It's obviously "yes". It's obvious that it could be done without breaking the laws of physics. Then other misconceptions easily fall away too. How much energy can be extracted? Now what swansont said is obvious, you would want a bigger propeller to extract more energy (the more you let the ground pull, the more you must push against the air). It's obvious that the moving ground could provide enough energy to overcome friction in a well-engineered device. It's obvious you wouldn't want a weightless vehicle, if you need a downward force against the ground to capture energy from its motion. Then, "How might that energy be turned into thrust, and does the device in the videos etc. conceivably do that?" is easier to think about.

I don't think the light has to be confined, and a system consisting of a glass prism with light shining through it should have more mass than just the prism. As a thought experiment, consider a massive particle at rest, and a photon moving with +x velocity. The photon has no mass, but considering the two particles as one system, this is not its rest frame. In the system's rest frame, the massive particle has some -x momentum balancing the photon's momentum. As a system, the particles' kinetic energy contributes to the system's rest mass. Likewise the prism+light's rest frame would be different from the prism's rest frame. I don't know if this is meaningful in general, since spacetime curvature depends on how the mass is distributed, and I can't imagine how to describe the effects of unconfined photons. However there are cases where it is meaningful, such as with a "kugelblitz", a black hole created by a dense concentration of light energy for example.

Yes, but gravitational potential is a Newtonian thing, and that applies (always) in a Newtonian analysis. I was going to use gravity assist / planet flyby as an example of how an object can climb out of a gravity well using less energy, in a changing gravitational field. However, in Newtonian physics, the planet's gravitational force acting on the object equals the force of the object acting on the planet, and the object still uses the same energy to climb, it just gets it from the planet. So it's not the case that a dynamic system alone breaks gravitational potential's path independence. GR is a different system, and I don't understand where and how the analogy to gravitational potential fails. But for example, I read that if you separate two masses, the GR analog to gravitational potential depends on if the masses are spinning, but the Newtonian gravitational potential doesn't.

A light-like path has a geometric length of zero, so all events on a given light cone should have a geometric (hyper)volume of zero. The interior of the cone would have positive volume (by choice of convention) or a time-like volume. The elsewhere would have negative or spacelike volume. The union of multiple light cones should have zero volume.* So for example if you have a light on the train, and turn it on at the beginning of the train's "life" and off at the end, then the geometric volume of all parts of the train that are lit over its entire life, is 0. If you consider only the lit part of the train (chop off everything outside the "light on" event's future light cone, and everything inside the "light off" event's future light cone), the train's entire existence has 0 geometric volume. Am I thinking about that reasonably? If so, I'm not sure if an invariant volume would make sense. It seems like a long-lived train, treated as a bunch of time-like world lines, should have a time-like volume. Or could you add up all the time-like world lines and get a different volume? * Or... did I make a mistake here? Maybe you can't just add up two light cones, because there are space-like intervals between events on the different cones, but then there are also time-like ones... Now I really don't see what a geometric volume could possibly mean.

I mean between two events. I'm reasoning that if two clocks are identical except for their histories (basically, same place, time, and speed), they must tick at the same rate, and have the same time dilation factor relative to some distant clock. Therefore the time dilation factor between two clocks can't depend on "how the clocks got there" (I'm generalizing), yet a calculation of something representing gravitational potential, could. I've never heard of the virial theorem. It looks not basic enough for me. I should look at the derivation but I'd probably give up after getting lost at the top of page 1.

But there is a unique single value gravitational time dilation factor between two points (in your example at least). Elsewhere on the web I see that gravitational potential represents the metric only as an analogy. Then the resolution might be that "gravitational time dilation is determined by the metric," while it's not always applicable that "gravitational time dilation is determined by gravitational potential"?

Resurrecting this idea, that I never ended up wrapping my head around... Say we use a mountain as an analogy for an arbitrary spacetime. There's a clock at the top. Two travellers each transport a clock down the mountain along different routes, and meet at the bottom. There, they generally find their clocks have aged differently, but the two clocks, together at relative rest, are now ticking at the same rate. Their clocks tick at a different rate from the clock at the top, but of course the two clocks at the bottom share the same time dilation factor relative to the one at the top. Therefore there must be a scalar number that relates the two locations, that is independent of any differences in how spacetime is curved along the different paths between the two. In this analogy, "height" works as that single scalar, and gravitational potential is meaningful. Is there another such scalar that relates two locations in an arbitrary spacetime, or is it just the "time dilation factor", and that factor isn't completely determined by any other scalar factor alone? Or, is it that gravitational potential loses meaning only where there aren't worldlines between the two locations? Or to ask in a different way, if you have two particles in freefall with different velocities, that pass through some one event and later pass at some second event, is gravitational potential certainly meaningful along their respective freefall paths, and if so is it the same along both paths, no matter how asymmetric the spacetime is?

2*sqrt(2) cm is the length of the square inscribed in the circles, which should make it easier to figure out.

I agree. Interesting that you reasoned it out right from the start, and transferred the events from the train to the tracks, at rest in their frame. Was that essential to the reasoning, or just because that's what the question asked? (If I could redo the question, I'd make them ships in space without the tracks, but would that make the question harder to reason?) Reciprocality should become pretty intuitive given the first postulate, it seems like a major prerequisite to understanding relativistic effects.

Yes, they're 100 m apart in the train's frame. The strikes / tunnel can't be 1 m in the track frame unless that 1 m expands to 100 m in the train's frame, which it doesn't. Yes, I used 'abstract' incorrectly. It's the added object (like a ruler, train platform, extra tunnel etc.), used to conceptualize the distance, that's abstract.

Err, too boring? Or not intuitive enough? How about, "What proper length of tracks is contracted to 100 m in the train's frame, if the train with a proper length of 100 m is contracted to 1 m in the track's frame?"

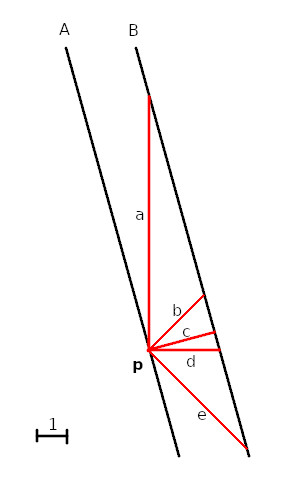
What is the length of a line segment that connects line A at point p, to line B? Is it one or more of a,b,c,d,e, or something else? If line A represented the world line of the front of a train, and B the world line of the back of the train, what is the length of the train? This doesn't even illustrate length contraction or the rules of relativity. I think you're missing some basic geometry in what you're asking.