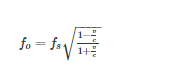michel123456

No. It was shown by Galileo Galilei in 1638. When you scale down (or scale up) you must take account of the square-cube law. https://en.wikipedia.org/wiki/Square–cube_law

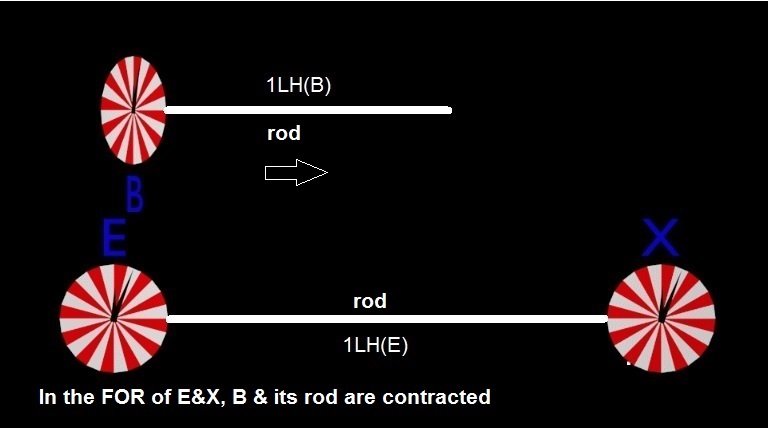
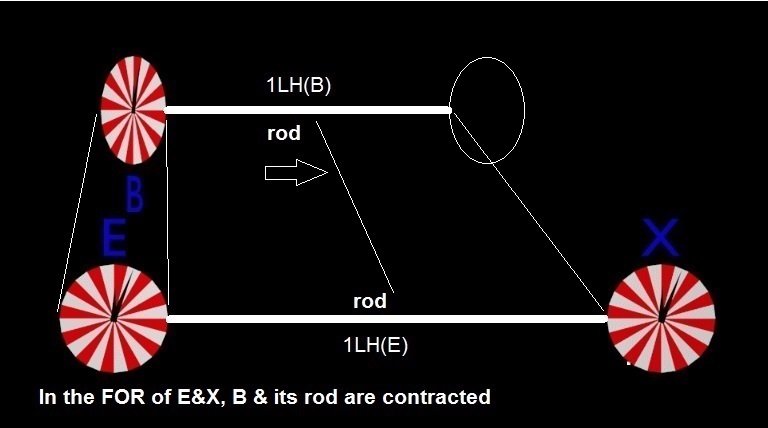
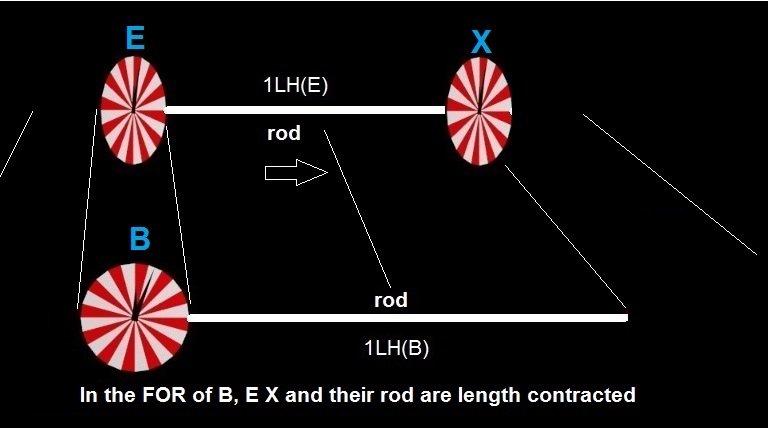
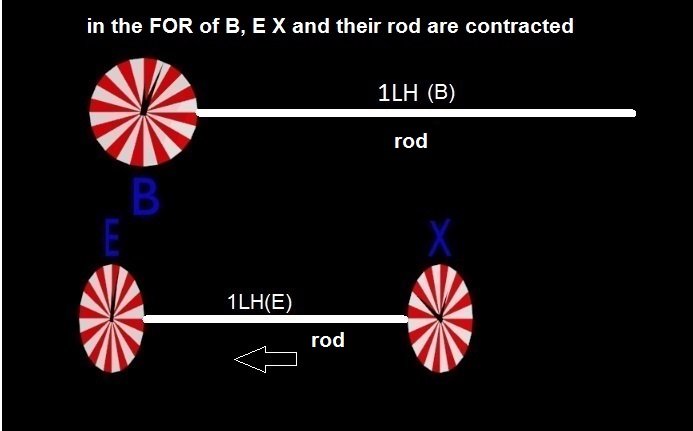
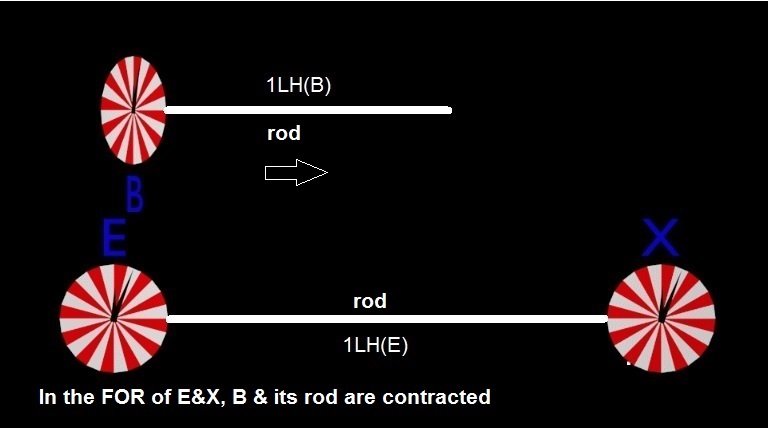
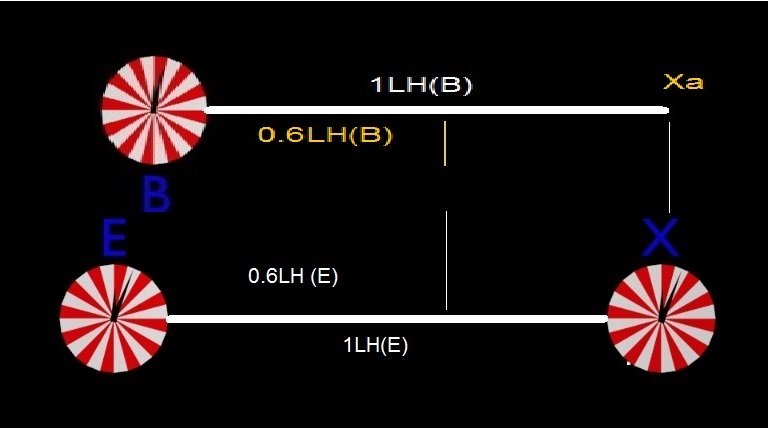
And here the solution. Diagram 1 is correct: diag 1. From E and X, because of its relative state of motion, B is contracted and its rod is contracted too. The effect is a scale factor along the line of motion: the units of length of B look to have be scaled by a factor of 0.6, because of the 0.8c velocity. diag 1 annotated The diagram says that B's rod that E observes as 1 LH corresponds to 0.6 LH in the frame of E. IOW the distance 1LH toward X (as observed in E) corresponds to 0.6 LH again in the frame of E. (and not in the frame of B) IT DOES NOT SAY THAT THE DISTANCE IS 0.6 LH IN THE FOR OF B. The 45 min calculated in several posts before does not correspond to anything. In the FOR of B, the distance is still 1 LH. And to travel 1 LH, the clock on B will tick for 75 minutes. Here below the situation from the FOR of B diag 10 It corresponds exactly to diag 1. The labeling has been reversed (E for B). In both, the distance to travel is 1LH. And in both, the time to travel is 60min / 0.8 = 75 min.

Think you for being constructive. I understand that some are getting tired, but for me also it is exhausting. It is so evident to me that the paradox is wrong that it blows my mind that it has been accepted and become a mainstream element of Relativity. The paradox must be wrong, not generally Relativity. One may accept that the traveler ages differently than the one ate rest, that is not an issue. But since velocity is relative, you cannot know who is at rest and who is moving (and all attempts to evade this point are BS- Bad Science). Since you don't know who is the one in motion, and who is not, the phenomena must be symmetric: what one observes is the same as the other observes , independently of Euclidian or other geometry. If you rely on the fact that geometry is not Euclidian to resolve the paradox, then you may be very intelligent but not very at the same time (like the twin younger than his sibling), another paradox. I think that's wrong. How do you get that X takes 45 minutes? If B starts at E, and the length to X is length-contracted to 0.6 LH (in B's frame), then X is already at that location (in B's frame) at B's time 0. Yes X is at that location, when moving toward B, it will take 45 min* to reach B. *the 45 min. have been calculated by others. I disagree with this.

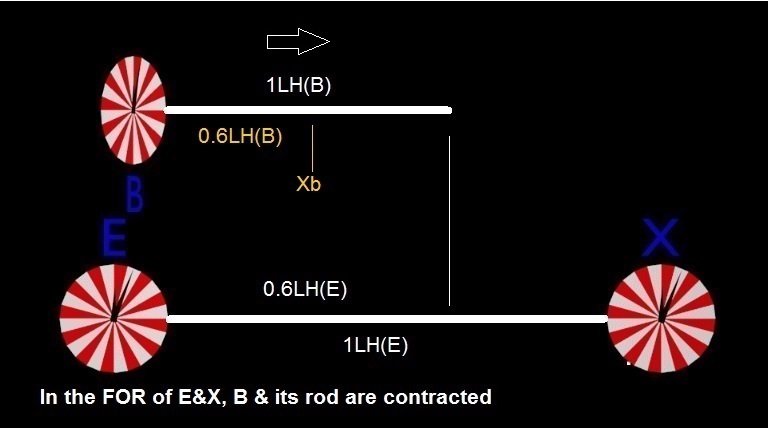
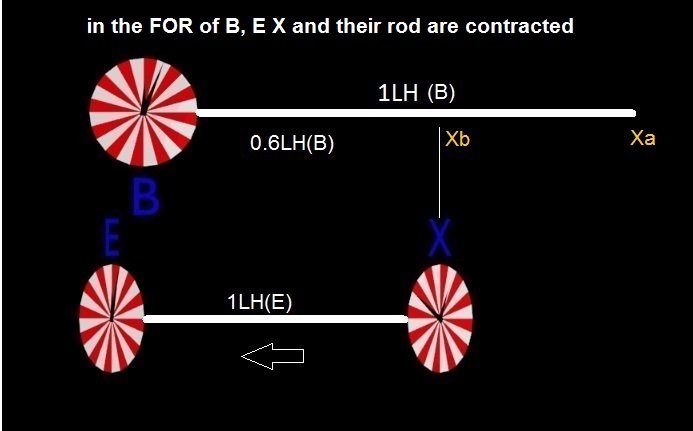
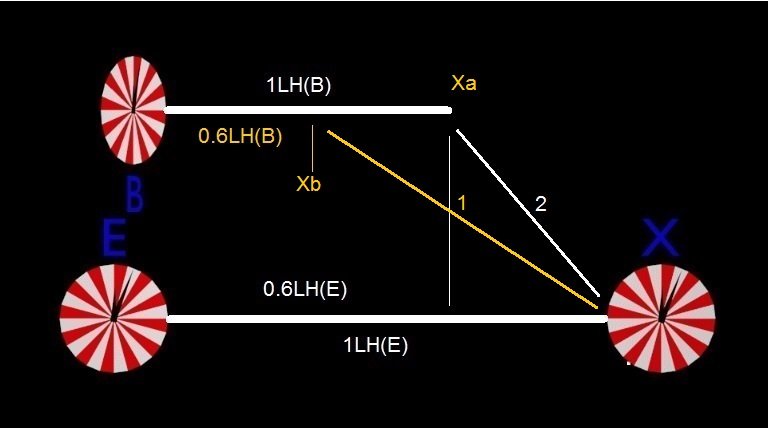
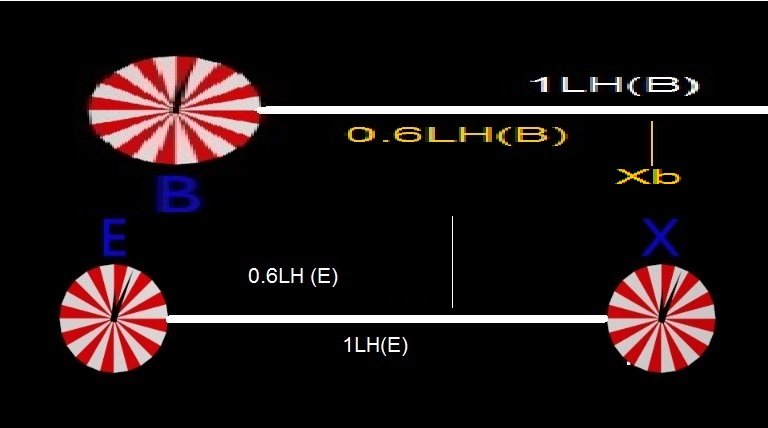
I have read this thread again and again However, all the answers from valuable members here are based on the assumption that I don't understand relativity. Exactly, it is not so difficult to grasp. Here below some new diagrams that I hope will make you think again. The basis has been stolen from the animations of @Janus on page 9. Find the error: diag 1 E and X are at rest, B is moving. I have inserted a solid rod 1LH long in order to evade the comments like "space does not move & thus do not contract" Since B is moving together with its rod, both are time dilated & space contracted. The rod of B is 1LH long in the frame of B (see below diag 4). The notation 1LH(B) means 1 Light Hour from the FOR of B. diag 2 Exactly the same diagram, with the position of 0,6LH which is the contraction of B's rod from the FOR of Earth and planet X diag 3 Exactly the same diagram with the position of 0,6LH in the rod of B (from the FOR of E & X). I have labeled the point as Xb, that is 0,6LH from B. diag 4 Now the things as from the FOR of B: E and X are moving together with their rod, thus they are time dilated & length contracted. diag 5. Exactly the same diagram with annotated the 0,6 LH traveled by E & X. diag 6 Exactly the same diagram with annotated the point Xb that is on the rod of B at 0.6 LH from him. X will reach this point in 45 min from the FOR of B. It is the same point Xb that is shown in diag 3. Xa is the label for the point at the end of the rod of B AND NOW THE QUESTION: diag 7 Return to Earth and planet X FOR. Where is the correct contraction? Is it along diagonal 1 or along diagonal 2? We can check out: Here with diagonal 1, the upper part has been stretched along the direction of movement in order to make correspond point Xb and planet X (because that is the result of calculations that say the travel of B will last 45 min in the FOR of B) diag 8 And here below with diagonal 2. diag 9 Which one is the correct one, where is the error?

But when @Eise quoted @Janus & explained the "However" part, that made sense to you, and it was about Relativity. As a reminder: Yes. B measures 75 min. Not 45 min. And there is no paradox.

I believe that in the FOR of B, X does not start to move instantaneously.

But that's incorrect. Times on B's clock don't add up to times on X's clock. Do you at least understand that you're talking about 2 different clocks? I know it's only page 12 but do you understand that much so far? You should read again the statement. It is about B's clock. It states that you must add to B's clock the RoS caused by the fact that E and X are not experienced in simultaneity by B.

Taking from your statements, reversed, that should give: 45+?=75 says "The amount of time that B's clock ticks while B travels, plus the time on B's clock when X begins equals the time on X's clock then B arrives." And we can assume that the result should be: ?=30min.

Thanks. Other question, on the grounds of what has been explained previously. I understand that there are at least 3 stages of calculations: Stage 1, Earth & Planet X are at rest, B is moving, contracted & time delayed : you insert the opening statements (1LH of distance, velocity 0.8c) and extract the result= Time of travel 75 min. in the FOR of E & X Stage 2, B is at rest, Earth & Planet X are moving: the distance between E & X is contracted by a factor of 0.6, so the distance becomes 0.6LH & velocity 0.8c give Time of travel 45 min. in the FOR of B Stage 3, B is at rest again, (quoting from Janus) “B is at rest while the Earth and planet X move to the left at 0.8c. the Earth, planet X, their clocks, and the distance between them is length contracted. B is not. It take 45 min by B's clock for planet X to travel from being 0.6 lh away to B at 0.8c Earth and planet X's clocks are time dilated and only accumulate 27 min during this time. However, due to Relativity of Simultaneity, the Planet X clock already reads 48 min later than Earth's clock when B and the Earth are next to each other. Thus the 27 minutes it advances brings it to 1 hr 15 min, as it passes B. The times on Earth's clock and B's clock when they are next to each other agree with the first animation, and the Times shown on B's clock and Planet X's clock when they are next to each other also agree with the first animation.” I have put these 3 stages in this sketch below: (this is not a spacetime diagram, simply a sketch that puts my ideas all together) Relativity of Simultaneity (RoS) is used in stage 3 in such a way that after the calculation pirouette, everything comes back in place: stage 3 corresponds to stage 1 with 75 min. recorded Time of Travel. That is fine. My questions are thus the followings, 1. why is there no use of RoS in stage 2? (the question mark on the sketch) 2.I am confused about the calculation procedure generally: why does it go one way (here from up to down) instead of going forth & back?

I am sorry. I am continuously messing the FOR's. B is at rest. Earth & X are moving. B is calculating with Lorentz transform what clocks on Earth & X are showing. At 12:00 the travel begins (that's the only thing I understand so far!!). By your calculations you say that when the departure bell rings at 12:00, clock on X shows 12:48. (according to B). Is that it?

@Eise here above. What is "Relativity of Simultaneity"? And what are the 48min that Planet X clock already reads when B and the Earth are next to each other ?

Time dilation factor is 0.6 Janus calculated 1h & 15 min dilated into 45 min. That is 75min x 0.6= 45 min. Lenght contraction factor is 0.6: the 1LH distance contracted into 0.6 LH So I expected that the time lag 1 hour (the delay) would become 60min. x 0.6 = 36 min. (the time light need to travel 0,6 LH).

Velocity is a vector: from wiki For E, velocity is speed 0.8c going out. The input, for him, is Vout=0,8c for X, velocity is speed 0.8c going in. The input for him, is Vin=-0.8c The direction of Vin is opposite to the direction of Vout: it is a different vector, so it must be understood as a different velocity. Which one is the correct for observer g? Vin or Vout? Which one is the "good one"? ------------------------- And I expected a result like 60 min x 0.6 = 36 min. I expected my calculation to be corrected by someone. (the one in the bottom that gives 36 min.)

I expected a result like 60 min x 0.6 = 36 min. Pretty straight forward yes, except the bold part. I am naive to believe that velocity is measured by δ distance divided by δ time. Since distance between the observer & the traveling clock remains the same (between the observer & the traveling object), δ distance is zero. So relative velocity (between the observer & the traveling clock) is zero. And the observer should see no time dilation & no length contraction (in relation to him). Although the same observer will see (according to what everybody say here) exactly what diagram 1 shows: a clock traveling 1LH in 1h:15 and ticking only 45 minutes, contracted sideways.

gamma is 1.66666 in our example. 1/gamma is 0.6

That is the formula for the Doppler effect for light. So it is about what you see. And this formula is only valid if the movement of sender and receiver is along the same line as the straight line between source and receiver. So here you have definitely dependency on the direction of the movement. I was just asking because from this formula was extracted what is happening (according to all other members here).

What is about the formula above? Is it about what someone sees, or about what actually happens? Again: Please explain the bold part: Please correct me: the planet X clock stays in X's FOR. Which means (by me) that X clock reads 13.00 when B passes next to E (for clock X, the distance is not contracted). Where do the 48 min. come from?

So you are saying that the 1h delay between Earth & X does not count for relativity?

So i have to rephrase my question: In animation 1 the clocks are synchronized and show the same time. If they show the same time, it means that it cannot be the situation in the FOR of the Earth. it is not the situation in the FOR of X either (there is an hour of difference: for the Earth departure time is 12.00 & it is 11.00 on X, for X departure time is 13.00 & it is 12.00 on Earth). The animation does not show the 2h15min that were needed for Earth to receive the signal of the arrival at X. IOW animation 1 is that of a virtual observer. Animation 2 has a different concept: it is from the FOR of B. So the question is; why is animation 2 not from a virtual observer? that would show the clocks synchronized I suppose, like animation 1. Sorry, I have to digest the answers. Yes i am baffled. To me, geometry shows evidently that the relative velocity is almost null since it is perpendicular to the line of sight. The fact that the virtual observer is in the same FOR of earth & planet X has nothing to do with that. Geometry still counts.

Why are the clocks E and X not synchronized?

.

Let's admit that there is a resultant velocity. Is this velocity equal to 0.8c?

I already answered that: And the observer is in the same FOR as E and X. Don't you see that E and X are standing still in Janus' first animation? That means the virtual observer is in the same FOR as E and X. Do you say that the speed of B related to Virtual Observer is 0.8c? But the distance between B and V.O. do not change. How do you explain that?

The observer is at rest wrt E and X, so it’s in the same frame. All of the measured values will be the same. You have evaded the question: what is the speed of B in the FOR of the virtual observer?

And what is the speed of B in the FOR of the virtual observer?