Arnav

Let's say i keep a zinc rod immersed in pure water as oxidation half cell, while a copper rod immersed in a 1 M solution of copper ions as reduction half cell. What then, will be the emf of the cell?

Consider a daniel cell. according to nernst equation, the emf of the cell is given as E = E* - (RT.ln([Zn2+]/[Cu2+]))/nF where E* = emf of the cell under standard conditions and the other symbols have their usual values. Let the concentration of Cu2+ ions [Cu2+] be 1 M. As [Zn2+], the concentration of zinc ions in the oxidation half cell tends to zero, the equation predicts that the emf of the cell will approach infinity. How is this possible? What am i missing?

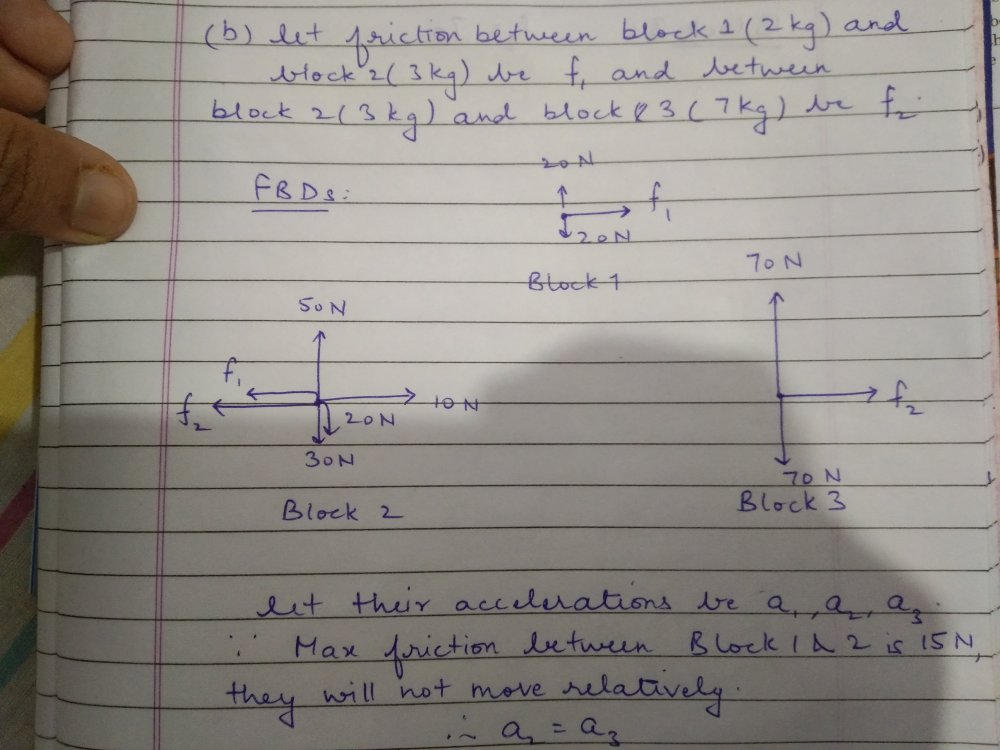
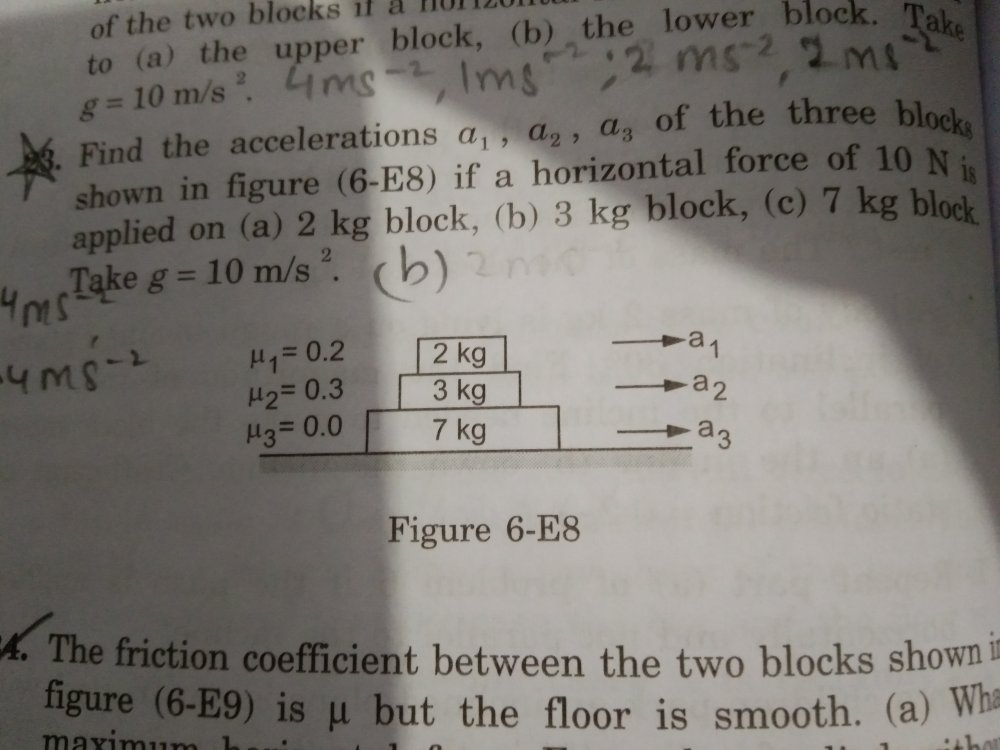
Maximum magnitude of F₂ = μ₂N₂ = 1.5g Maximum magnitude of F₁ = μ₁N₁ = 0.4g yeah i understand However, in a single case, lets say 10 N force is applied on block 2, i would be having 3 FBDs, each corresponding to one of the blocks, which would give me 3 equations involving five variables, f₁ and f₂ (as i dont know if they are limiting or not, and f₃ will always be zero as the friction coefficient is zero so not counting that) and the accelerations of the three blocks a₁,a₂,a₃. I am confused how i go on after that. I obviously need at least the same number of equations as the number of variables.

Yo man you just cleared my head completely! Thanks! I really confused whether to give up the principles of VBT while studying MOT or not, but thanks to you it's clear now Will definitely check the website

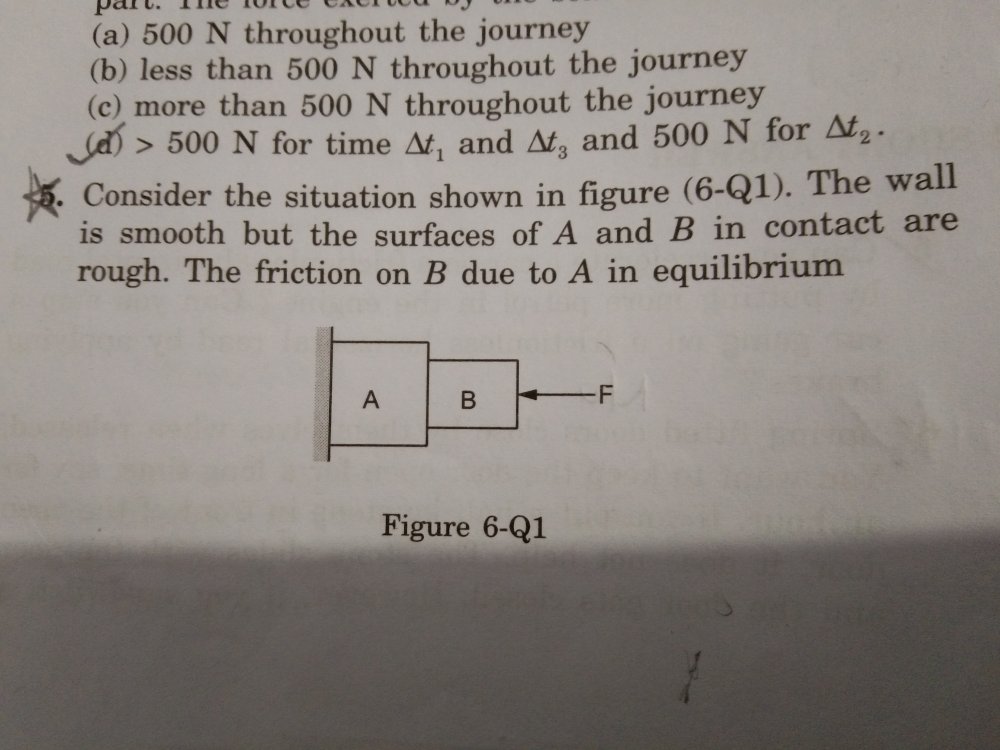
here is the rest of the 5th question, the correct answer is given as the 4th option i think by equilibrium the author means to ask whether the force force F on itself,alone, will it be able to keep the blocks in equilibrium?

Here is what I have done so far I am getting 3 equations and 4 variables. When i see a solution of this question, it is said that blocks 1 and 2 will not move relative to each other as well. I dont get how i can conclude that f₁ and f₂ will be such that all three blocks are not in relative motion. Do i take into account the self adjustable nature of friction? or am i missing something else?

@studiot do you mean that the magnitude of coefficient of friction, the weight of the blocks is not given? or is the question incorrect?

I tried to, but i could not come to the conclusion whether the friction between block b and c will be able to restrict their relative motion or not

How do I do the part b and c of this question (23)? Any hints? One part of me says that in part b, the max friction between the 3 kg and 7 kg block is 15 N, so there will be no relative motion between them. What about 2kg and 3 kg block?

I am having trouble understanding this question. The questions asks the direction of friction. The correct answer is the is that the system cannot remain in equilibrium. One more question is that if the friction coefficient between A and the wall had not been zero what would have been the direction of friction due to A on B then?

A basic doubt in molecular orbital theory. While writing the electronic configuration of molecular orbital, for example say of Fluorine molecule, which forms a single sigma bond between sp3 orbitals, we start the configuration with sigma1s² orbital. Does this mean molecular orbital theory considers that when atoms combine, all of their orbitals are fused? Even the ones not participating in the bond? Or does it consider that the bond between atoms is not just a result of the bond between valence orbitals, but all of the orbitals of combining atoms? I have only been introduced to this theory qualitatively, so the questions may seem a bit weird.

A question in a very famous physics book in my region is as follows: While walking on ice, one should take small steps to avoid slipping. This is because smaller steps ensure: a) larger friction b) smaller friction c) larger normal force d) smaller normal force This is a single correct multiple choice question. Contrary to common idea, which is that smaller steps would give you more friction, the book states the opposite, smaller steps would give smaller friction!? So as per my reasoning, in both the cases, the normal force would be the same, equal to mg, where m is the mass of the person, to maintain vertical equilibrium, so options 3 & 4 are incorrect. Now while taking smaller steps, the impending motion of either of the person's feet relative to the ice is very low, as compared to the impending motion of the feet relative to ice in the case of larger steps, which should be more, so that gives lesser friction in smaller steps( less than the limiting friction), giving the 2nd option as correct. Is my reasoning correct?

Many indian books have that term...not under the topic of historical development of periodic table, rather under the topic modern periodic table and the broad classification of elements in it. They state that s,p blocks are called representative elements and don't give any reason

Oh nice 🙂

okay thanks guys. I dont know why the books in our education system still use the old terminology like representative elements😬