-
Posts
259 -
Joined
-
Last visited
Content Type
Profiles
Forums
Events
Posts posted by Sato
-
-
Just the amount of background knowledge has increased, say as compared to say two hundred years ago. Further and further specialisation has made it more difficult for people to read science and mathematics papers, this includes experts in one field reading something in another very close field. Working from just high school mathematics and science is not enough in general.
So people have to read a lot more and so on. This should be easier via the internet as you have said.
As I said in my first post, an argument against examples from centuries ago is reasonable. But that's not what we're discussing; the examples of successful scientists (mathematicians) I gave made contributions in the last 50 years, not 200. And of course we are not talking of people with only high school maths/science; everyone referenced took the time to learn very deeply into each field.
I also elaborated, positing that work, such as Grothendieck's even 60 years ago, was as complicated as the work of a given researcher today. This is not an assumption or based purely on intuition; I've seen modern, rigorous research published by tenured professors that's conceptually "simpler" than the content of some higher level expository texts.
In sum, everyone I've noted had to study material approximately as specialized as they would today, where we define "approximately" to mean that any added difficulty is mainly mitigated by the technological advances we discussed, as well as by the significant decrease in poverty and increase in household prosperity since (w.r.t. the countries of our examples). This I'd say would incline the "net difficulty derivative" to a negative.
Nowhere have I said it is impossible to make contributions. Just today is is less likely and I think it will get harder.
I know, you've made this clear. The qualification was being impossible "...unless they have some 'extraordinary' gene", as you were asserting by discounting certain examples as extraordinary and not to be taken as evidence. This I can accept for Ramanujan, though I'm not so sure how true the lore is, but you repeated it for Hua, and under a lenient eye you may have continued to do so for every such individual.
I think in my response to the previous snippet it is actually more likely and has become easier. I reiterated and added some points, addressing your concern with the increasing specialization. (Where) Do you see the net difficulty increasing, given those points?
Note that we consider "increasing" up to some reasonable future time. Just as a million tonnes might be added to the literature in the next hundred or so years, positively requiring more formal training, new learning technologies and growing economies, whether Salman Khanian, Star Trekkian or pharmaceutical, may be brought out that mitigates this. Else we may have 30 year PhD's, and those contingencies aren't in the domain of this discussion.
So, excluding maybe some very famous examples, I suspect it will be difficult to point to many amateurs today that are making real contributions. Not none of course.
The examples I know of are not very famous examples, and you'd be hard pressed to find their names in this context of "amateur" scientists. Namely because each of them kept a stay in the community, administrated scientific programmes, received funding, and weren't so sensationally idiosyncratic as Ramanujan was. This SFN thread might contain the biggest listing of "amateurs", who've made modern contributions of that magnitude, on the internet.
The individuals I personally knew, though I do not know if you'd count them as scientists or engineers, are similarly not on this radar. Still, they've received public and private funding, held visiting positions at top US institutions (according to a recent search of him, the policy of the universities is that you ought to have a PhD plus some experience to be a visiting scientist... or equivalent experience), and one (the applied physicist) has his developments being contracted by hospitals. I know of more amateurs, at least in physics/maths, who made such contributions and did receive funding, than I do those who did not. This is an offshoot of Arete's response and your agreement with him.
Surely not many, surely not many who advertise their circumstance, but surely a paucity of people of some quality doesn't mean that quality should be discouraged. Of course, how likely is it that someone will be more inclined to independent study than university, and will do so enough to make contributions? Unlikely, but if they are and they do, they should have a place.
So your friend has published original work?
The physics student is not a friend of mine. I met him at a public lecture (not the kind of public lecture you might be thinking of) and he discussed his efforts a bit. I have his phone number and may ask for lunch so will bring it up if I do. Even if he has published original work, I think he would fall into this category of people with no formal training in their fields that we are discussing.
I thought we were discussing people who are or wish to produce original work?
This maybe the route of our seeming disagreement.
I'm not sure where I contradicted our point of "people who are or wish to produce original work". This what I think we are discussing.
I do see one latent point of disagreement, maybe delineating it will help. I do not think that working with the guidance of a professor removes our label—an interesting edge case is if the professor is himself an amateur. Secondary school students not so unoften publish work in collaboration with researchers; this is more common in specialized schools in the US I think, but I would still call them amateurs. In fact, I think each of our examples, in addition to the independent study and research, regularly communicated with professors and received advisement. What makes them such is that they didn't go through any formal training.
Otherwise, wouldn't it then be necessary to acknowledge anyone without any formal training, as long as they had some consistent communication with an academician about their research, as a professional themselves? And if we do this, I think we lose our notion of amateur, and gain one that is incongruous with reality. No matter how many hours spent speaking with a professor, it will still be more and just as difficult for one without the credential (say, the PhD) to be acknowledged by the community as a professional.
So, provided your friend convinced a potential supervisor and the graduate admissions board that they could complete a PhD in reasonable time then they maybe admitted. The best way to convince them is with formal qualifications.
I've seen a professor speak of doing this as "decreasing the market value of a degree, which my career relies on". Perhaps you or the Polish school may be okay with this, but I think there is harsh sentiment most elsewhere. For the sake of making this thread a repository of "examples of idiosyncracy", as I've stumbled on a handful, I ought to mention Demetrios Christodoulou (who went to university with a past "advisor" of mine, from whom I learned that apparently Wheeler ran a program just to bring Greek secondary school students to the physics dept in this way), John Moffat (by way of Einstein and Bohr), Stephen Wolfram (in particle physics, before his well known excursions), Paul Lockhart, John Sterling, Ovidiu Savin, Jamshid Derakhshan, Aleksandr Khazanov (our required "early and tragic end"), and Jake Barnet (who was an old friend of mine, now on with his PhD; I spoke to him a few months ago, and he said that they actually agreed to and did award him a BSc, which may be a source of scarcity).
They guy with the credentials, all other things being equal, would be seen as the safer bet. This applies across all aspects of human interactions and not science in any particularly special way.
I did not say all other things being equal, I said it would not even be looked into if all other things were equal. That is, without consideration of independent research, a publication, or even extra study, the person would be looked over. I think this is unjust and assert that it is generally wrong. Fortunately there are some motivating examples in this, as enumerated above, but there is a pattern that those who took them on were stars in their respective fields, not representative of the majority's views and positions. Perhaps it is because they have more pull, but in any case, their potential to achieve runs with the luck of who they meet.
No papers I have read have been produced by people genuinely outside of academia.
...
Still, I do not think many amateurs have great impact across science today.
That may be true, and it is precisely because there aren't many of them, and I discussed this above in response to snippet #3. Our examples, the individuals we've found to satisfy this quality (I am a bit disinclined to the word "amateur"), have consistently made significant contributions in their respective fields which still echo today.
The two acquaintances I referenced won research grants, beating out the applications/proposals of even university labs I'd imagine, so there is certainly some impact being made right now. Of course this point is moot if you do not count engineers as scientists in this context, but this is the same work that many "applied" scientists are doing today.
Addendum:
I've read on forums complaints from engineers who work with technicians who'd, after years of work for the company, been promoted to their ranks. They rightfully complain, because apparently those "engineers" aren't able to do the necessary mathematical modelling and computations, and don't have the necessarily precise knowledge that they do. We are not discussing these types, we are discussing the "academic amateur" types who do study all of that.
0 -
One reason is simply that the body of knowledge is huge. People need to specialise to a certain field and then pick a few selective topic therein. Because of this someone with reasonable general science/maths knowledge will find it hard to contribute.
There are some subjects that seem more amenable to amateurs. Tiling theory seems to be one. For example, Marjorie Rice found certain tiling of the plane that were thought not to exist. So again, amateurs can make contributions, just I think generally it is unlikely and getting harder to do so.
We are discussing individuals who do specialize in very selective topics. Not science enthusiasts whose study time is occupied by PopSci magazines and Nova Documentaries, but individuals who took great interest in some topic(s) and put the work in to contribute to them.
Luogeng and Chaitin both made their contributions around the time of Marjorie Rice. I've also just recalled another name, Walter Pitts, who did as well. They all did significant work in very deep and difficult fields, but I can't say I've heard of any amateur tiling theorists besides Marjorie.
I do not see how it is much more difficult today; maybe they'd have to read an extra monograph and 50 more papers than they did then, but this doesn't add much to the unlikelihood factor. Today I would actually assert that it's much easier, given the plenty of textbooks, lectures, and explanations available freely on the internet. Maybe even more importantly, it's much easier to contact professionals in the field to ask questions, and even collaborate with other students. No waiting weeks for a letter, digging for an Alaska phone book, or trekking to find someone willing to help understand some exercise or concept: you have email, a direct listing of an author's research interests and their other publications, IRC, forums and stackexchange where I've never not received the proper help. I think this trumps the extra reading they'd have to do for the more developed field.
And in precaution, I will reiterate that the work of a researcher could be and was just as complicated at their time as it is for many today. Surely you're familiar with Grothendieck's work in the 60's, as an example closer to home. The aforementioned individuals did work whose complexity was not so far from today's, and did so with greatly less resources.
Do you have any rationale for it being more difficult today that stands against this point?
In the way of this collaborative discussion, given this, I think the conclusion is that it is much easier, and accordingly that it should be discouraged less—following in your trend of relating likelihood and difficulty.
I disagree with this. My argument is more like the following. Unless an athlete trains hard, follows the advice of the coach, eats well, enters competitions at various levels and so on he/she is unlikely to be selected for their country's Olympic team.
In science the standard route of training is through academia.
I do not know if you intentionally distorted my comparison to athletes and genes for the sake of debate, or if you misunderstood my wording, but I was countering your comment regarding Hua as "extraordinary" in the Ramanujanian sense. That one cannot make contributions without a formal education unless they have some "extraordinary" gene, but as you acknowledged in your last response "...the individuals listed put lost of time and effort in, coupled with some inherent talent and a bit of luck they made contributions.". These are qualities that are not so rare in whatever circumstances, and so my point was that saying "don't reference person X because he is extraordinary" isn't a very reasonable way to discount them as "evidence". I thought it was clear that I was discussing a gene for becoming a self-taught scientist, not a gene for becoming an academic.
He is now taking physics courses at University. That is great and lots of people study part-time or online etc. It is difficult to organise time for this, but people do manage.
Has this guy published any original works? And if he did, would we really call him am amateur if he has some university training? Okay this maybe at undergraduate level, but still.
He studied most of the undergraduate curriculum and many "graduate" topics himself (actually with a textbook-club of amateurs he started). I think it was that were some topics he didn't cover so thoroughly in his independent study and weren't too pertinent to his interests, that he's decided to take a course in them before applying to grad school.
I am not sure, but if he did, most of his training, waive maybe two courses, was independent. Would you really mark someone university trained for that, especially if they were courses auxiliary to his interest done primarily for the sake of boosting a C.V.. I do not think that's a proper threshold for "university trained", as even your previous responses implied.
*discussion of people who crossed over, from my examples to Witten*
There are other examples of people 'crossing over'. It is considered very difficult, but clearly possible.
Anyway, I think this is twisting the topic slightly. The examples of people I know who are professional scientists may not have an undergraduate degree in exactly the field they now work in, but they all managed to complete a PhD programme in a closely related field.
What would you say if our aforementioned physics student, 20 years prior, did not major in English. What if he studied a semester of physics and left for work or some other circumstance. What if he was in the same exact circumstance he's in now, having not been awarded a degree but certainly prepared?
I think that you, and I posit that this is a terrible thing, a form of discrimination if you will, would deny him the opportunity to enter the community even if he did produce his own research. In fact, and even more acutely, I anticipate that you would deny to train him and even let him go through that 'trial by fire' that Witten went through, simply because he does not have even the lower credential. That, seeing him beside another who did have a credential, you would not even consider the former, would not give him the opportunity. I am almost certain that this is true, and it is a sort of litmus test for whether the concern is for the credential or the ability. My point is that it is wrong, morally and economically, to weigh his efforts lesser than the other, likely not even considering who you might infer to be a stronger scientist. You can consider this by induction up through faculty positions.
Here when I say 'you' I mean the greater academic-science community, but I imagine you would do similar placed in those circumstances. This, I think, makes it clear that the credential and not the content is the concern.
0 -
You may be able to find a few examples of 'amateurs' who have contributed. Both Hua Luogeng and Srinivasa Ramanujan may fall into this category, however giving a few examples of extraordinary individuals who lacked formal education is not really supporting the idea that 'amateurs' are likely to be able to contribute. These people were extraordinary and not the norm.
It is quite possible for someone to contribute, especially with the arXiv and open access journals. However, experience suggest that few 'amateurs' really produce work of great interest to the community. The problem is it takes a lot of time and effort to study mathematics and science, not everyone has the time to do this on top of other work commitments. The same is true of genuine original research. On top of that, experimental sciences may require equipment that is not available to 'amateurs' with modest budgets.
This is precisely why I didn't mention Ramanujan, because of the lore of savantism surrounding him. Hua may be likened to him by their shared poverty in youth and both receiving no formal education, but Hua didn't hint much raw, extraordinary intelligence in the sense of Srinivasa. He took interest in mathematics, studied, read papers, picked up on some ideas, and initially published some competent (perhaps not significant) work and corrections in a local journal. Chaitin went to an advanced science school nearby me and certainly didn't stand out, entering an okay city college afterwards.
These are not extraordinary examples, these are examples of individuals who took on independent study and entered the necessary effort. I imagine you come across many enthusiasts who try to propound their own ideas w/o much awareness of the field, and so result in either crackpot or trivial work, but those are a different breed. The argument you are using is the same one as telling an athlete "you don't have the genes", and you can be sure there are many ashes-to-phoenix stories of this. Mathematics even, is less dependent on 'genes' and intrinsic extraordinarity than athletics, so this should be much less of an issue. Of course, we are talking about the ability to make contributions, not necessarily great ones.
On the topic of not having enough time, I recently met a man, he looked like he was in his 40's, who had studied English in college. He'd been working as a financial controller but simultaneously independently studied enough to learn QFT, was preparing for the Physics GRE, and was taking a course at a university round here to bolster his C.V.. I know someone who studied physics, taking no pure math courses past linear algebra in college, and is now doing research at a big place here in the USA, in what I can barely recognize as algebraic topology. Neither've these examples had any training in pure maths, but to sharply different degrees; what would you say about them? Is your inclination to formal training in the field of interest, or just a credential?
0 -
I think that it's a bit (very) myopic to say that it's unlikely for someone without any formal education to be able to contribute to science. Sure, you can say that it's unlikely that they will contribute to science, as most people who intend to go to school, but certainly you can't say much about their ability to do so. Not having a formal education does not equate to working in isolation. I've contacted and been given regular advisement from professors in both the Ivies and a local college, while not being a university student.
It is reasonable to say that in order to contribute to modern research, one must train at a particular pace and in a particular way, particular in that it isn't compatible with how things were done centuries ago. So to some extent, the argument against allegory to Newton through Aristotle is acceptable. But, there are much more recent examples. Take Hua Luogeng, who through the later half the the last century made several notable contributions to number theory and related areas. More than that, Hua eventually came to direct and pioneer several major efforts for the mathematics research community and maths education in China, even heading the development of relations with western scientific institutions after political turbulence in the 1960s-70s. Take another example, Gregory Chaitin, who co-discovered and heavily developed algorithmic information theory, a field both thickly profound, rigorous, and even applicable in statistics and machine learning; maybe one of the most recent advances in science that I'd say so. Gregory is still alive and doing research, favourable in this respect to thousands of apathetic maths/CS faculty round the world, today. These people both ended up accepted in their communities and became full professors, not that that fact is necessary to realize my point. There are several others like this that I've stumbled upon, but these two I thought of immediately; I'm not sure that there's any enumeration of such people around the web.
To add personal anecdote, I've been acquainted with an applied physicist (full with DARPA funding and full knowledge of PDE's) and a biomedical engineer (currently a visiting scientist at a top institution), who haven't more than a high school formal education.
Would you have it that these people not done the good they did, so long as they went the usual way? The only reason they succeeded is because they stumbled upon individuals who cared more about what they could do than what credentials they ought to have, even before they made their marked contributions. This right now isn't directed in response to ajb's comment, for I don't know his views on this or if they're mutable, but I wish vainly that anyone with such frankly bigoted views might come to know this and end up not denying someone who seeks opportunity the hand they need.
I've not had a good experience with formal education and am currently deciding on whether to pursue it the coming fall, but the rash of meta-ivory tower bigotry that I find is strongly disinclining me. Whether this is reasonable is a murky matter, but I feel very strongly about the freedom to pursue a life of scientific contemplation (in analogy to religious/spiritual contemplation), and am not keen to a compromise that siphons away the substance and presents the veneer. Who would *dare* disparage a jew for trying to practice his religion, even though it would have made his/her life much easier to have adopted a slightly different mythology and switch the star for the cross; this is the retrospectively irrational decision my grandparents made in those circumstances.
Not everyone fits into the formal institutions of training and education, and they may flourish if supported on the paths they take. It is scary that they are often not. I haven't yet been put in the circumstances where I'd need serious support, but the telescope stares harshly. I hope this clarifies some ideas that may not be so obvious, or their seriousness not so obvious, to those who have not had think much about this trouble.
1 -
I'm pretty sure I've read about pocket sized spectrometers a few years ago, if only for industry applications. But this one looks like it's to be commercial, which worries me that they dampened to quality, but very cool otherwise.
Here's a somewhat relevant paper about using a laser pointer to build a small, low-cost raman spectrometer, http://sites.northwestern.edu/vanduyne/files/2012/10/2004_Young.pdf. May be of interest.
0 -
I find that having a rational motivation for my work makes me more productive.
It's very easy to rationalize doing anything, and once you've got the idea "the thing I'm doing right now is important because ... and it's interesting because ..." grokked so that you can access it for an instant every few seconds, you should see yourself more fit to focus, consistently.
I think this applies widely, whether to a field worker feeding his wife and children, or a mathematician grasping the all-pervasive concept of categories, finding (or tricking yourself into feeling) interest and importance in the work is usually doable.
Not that ajb can't feed his wife and/or and grasp mathematics at the same time
 .
. 0
0 -
Hi there,
One comment, not really relevant to the content, is that you may want to substitute your references to wikipedia articles with references to some expository textbook (like EoIT) or surveys describing uses of Huffman and Arithmetic coding. There's usually actually such references on the Wikipedia pages themselves.
Otherwise I'm not too familiar with the usual approach, but your work looks interesting!
1 -
Hey Daedalus,
I'm incredulous!
It really is great.
Do you compose it raw or is it a mix between yours and existing rhythms and melodies?
There's something faintly familiar about it.
0 -
It looks that many of our resident helpers aren't aware of the fact that, most of the time, the forum-theorist isn't interested in the actual science. They have read and watched Michio Kaku and Nova, contemplated Neil deGrasse Tyson's videos on the big bang, and read Brian Greene's explications of the eleventh dimension, and that is the kind of thing they associate with science (in the vein of what Strange said).
The big misconception seems to be that they have any interest in learning science. To the same extent that a film like Catch Me If You Can gets me interested in accounting, or any movie about financial fraud might incline me to forensic accounting, scifi and popsci inclines them to science. In those circumstances, they might find integrating functions in their intro to kinematics section just as fun as I'd find diagramming the distinctions between single and double entry systems.
It is more important, I think, to verify that they have any interest in actual science, by showing them actual science.
I have a friend, very smart and very creative, who wanted to build a system that could use electrical shocks to boost mental agility. His goal was to make a product out of it. He'd seen such devices in the market, and I asked him some details. He described to me that he built it around a pair of sunglasses, so as to make it mobile, using some sponges, wire, etc.; it was a makeshift that'd be found in an eHow "Make your own transcranial electrical stimulation device". I pushed and said in order to get funding to make it a viable product, he'd have to edge on making it smaller, more efficient, and more precise, and noted that lots of the current technologies can do it. I linked him to some related wiki articles on EE and some studies on different kinds of transcranial stimulation, advised that he should mathematically model his builds before spending the time / money building them, and talked about the digital signal processing and signal transmission science he'd have to study to actually build the software system.
After about a month he announced to me that he just wanted to be a designer and the idea guy, and would hire engineers after he got funding. He spends lots of time thinking, scribbling ideas into pages and pages of his notebook, but it's a different kind of thinking. He also has vices and hobbies and work that, whenever he isn't thinking these exciting thoughts, will take precedence. I think this is the case for most "cranks" who come on SFN, and I think this is incompatible with actual scientific study. That is, the actual work involved requires more than a bit, very possibly an intrusive bit, of dedication and motivation to move forward in.
1 -
This isn't the solution that came up on WolframAlpha, but maybe it's equivalent?
Take a look, maybe it'll illuminate something: http://www.wolframalpha.com/input/?i=c+%3D+%28lambda%2Fu%29*e^%28-%28u-lambda%29t%29+solve+for+lambda.
Note that the [math]W_n[/math] function is this http://mathworld.wolfram.com/LambertW-Function.html.
1 -
The courses you take should be much easier than the work you do as a software engineer. As a CS major you'll probably have several requisite courses, and I think my assertion will be made clear by drawing a distinction between how you learn in some of those, and how you use what you learned in production:
- Programming: In college you'll be given a programming language to learn and a set of standards along with it. The lectures will basically be a series of tutorials, teaching you how to take ideas and implement them as programs. You'll learn the common lingo of programming languages and the associated concepts along with it. Your problem sets will be exercises in expressing mostly cookie-cutter systems as computer programs. However, in production, you'll likely have to learn to use different programming languages and technologies without the direction of a curriculum. This is even more striking if you're inclined to work with logic or functional programming, which is a whole separate set of concepts from what's usually taught in schools, imperative programming. And much of the work you're given will not be cookie-cutter clear; the person who wants it built might not be able to express what they want in a way that can easily be translated into a program, so you'll have to infer the missing pieces yourself; sometimes you'll have to implement obscure heuristics to get something very specialized done, that won't match anything you've learned before; importantly, you might be working in a team, and you'll have to learn and conform yourself to a new set of standards and practices, and put effort to make your code readable in a way that every technical member of your team finds reasonable.
- Algorithms and Data Structures: When you study these topics in college, you ought to be taught in a somewhat rigorous fashion. If this is the case, it might be your first encounter with something similarly abstract. You'll be taught the all of the concepts, which initially may seem a bit difficult and disconnected, but will be understood after a few examples. Past that exposition, you'll be taught and expected to memorize various widely used data structures and algorithm schemes. Then, you'll be taught and expected to know the efficiency (complexity) of certain popular algorithms, as well as a bevy of trivial ones as examples and on problem sets. Once you enter the industry, though, you'll be given long and often unreadable clumps of code, and you'll be told to optimize them. Sometimes you'll be told to document the efficiency of the old code and your revisions, and to do this you'll have to read and understand every significant bit of it. If you're only told to optimize it, your team or employer's only tool being a benchmark, you may be able to skimp by without doing any actual complexity analysis; but applying stock heuristics won't always work, and you'll then have to read through, understand, and apply those ideas from complexity analysis to comparatively non-trivial code. If you're assigned to write an algorithm and/or data structure that meets some speed/storage/efficiency specifications, you'll have to design it with everything discussed so far in mind, wary that an inefficient implementation might stall, break, or even bring down a server! If you're told to implement some already existing algorithm, you'll have to understand it well enough to implement it properly in whatever programming language you're using, knowing the ins and outs of your platform and optimizing the implementation accordingly (see previous bullet).
Along with those, there are many things you'll be pressed to learn on your own on the outside, from using development tools like git and vim to setting up heroku servers and maneuvering the facebook API.
You can be a successful programmer without using much of these "industry skills" though. Mostly in web development and simple business/records software, things can be built nearly as easily as college exercises can be solved. In order to strive upwards and achieve in the field, however, it is necessary to put in the effort and acquire those skills and competencies as outlined above.
Good luck with your ventures!
1 - Programming: In college you'll be given a programming language to learn and a set of standards along with it. The lectures will basically be a series of tutorials, teaching you how to take ideas and implement them as programs. You'll learn the common lingo of programming languages and the associated concepts along with it. Your problem sets will be exercises in expressing mostly cookie-cutter systems as computer programs. However, in production, you'll likely have to learn to use different programming languages and technologies without the direction of a curriculum. This is even more striking if you're inclined to work with logic or functional programming, which is a whole separate set of concepts from what's usually taught in schools, imperative programming. And much of the work you're given will not be cookie-cutter clear; the person who wants it built might not be able to express what they want in a way that can easily be translated into a program, so you'll have to infer the missing pieces yourself; sometimes you'll have to implement obscure heuristics to get something very specialized done, that won't match anything you've learned before; importantly, you might be working in a team, and you'll have to learn and conform yourself to a new set of standards and practices, and put effort to make your code readable in a way that every technical member of your team finds reasonable.
-
What exactly is mathematical physics in contrast to non-mathematical physics?
I would imagine it is physics done in the way of pure maths, as in designating axioms, definitions, some inference rules implied by the context, and then deriving theorems about the subject matter, or similarly constructing structures that correspond to physical phenomena. But then what is the difference between that and, say, solving some equation and deriving the result using the informal tools of calculus/algebra. Each step in the derivation might not be given a justification like a proof step, and there may be differences in formatting and fullness, but wouldn't it be the same application of the theorems of algebra, analysis, and geometry? Is the only difference in style?
I asked this to an astrophysics professor two years ago and he said (paraphrased) "it's just physics done more mathematically". At the time I think my impression was that mathematical physicists used more obscure mathematics, so after asking if they also used something like, say, differential geometry, he answered that both types of physicists use it. I don't think I understood at all what he was getting at then, but think the idea above matches that, but am still unsure so wondering.
Thanks,
Sato
0 -
Many of the courses you'd take for those specializations would be general prerequisites, like introductory chemistry, organic chemistry, biology, earth science, physics, calculus, etc, each of which would usually take two semesters on its own. So, by the time you finish taking all of the low-level stuff that isn't your particular interest, you will have taken up two years, not spent money on courses that are very similar between universities, have better chances of getting into the university that you want, and then be able to take primarily courses in your specialization of interest. If you don't want to or can't continue on, rather than dropping out as a sophomore with lots of debt, you now have a relatively inexpensive associates degree in a science. It'd probably be best to speak to a professor or department head at the community college you're looking at about selecting courses for this purpose.
Hope that helped!
1 -
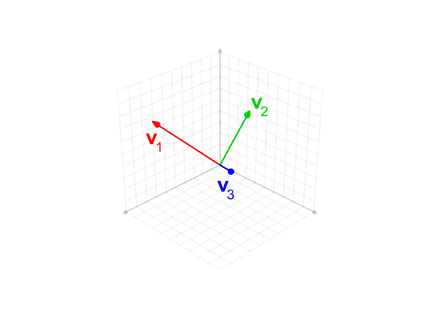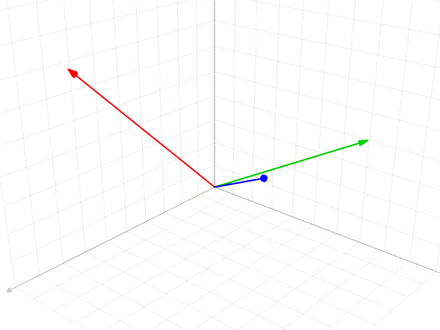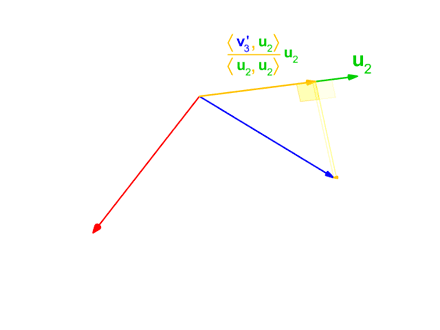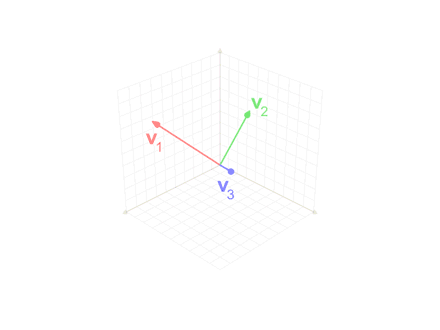
The values in a vector can represent many things, but most likely you're dealing with euclidean vectors, and so you can take them to represent positions in space. Each element then represents some distance along a spatial dimension. For example, you can take the scalar [math]x[/math] to represent the distance along just one dimension, such as length, height, or depth (canonically [math]x[/math] is length). If you have a two element vector, you can take [math]\begin{bmatrix}x \\ y\end{bmatrix}[/math] to represent some magnitude in length, and some magnitude in height; that is, imagine it points to some place on a 2D plane, a little to the left if [math]x[/math] is negative, to the right if [math]x[/math] is positive, or in the centre if [math]x[/math] is 0. Similarly, it'll point lower if [math]y[/math] is negative, in the vertical centre if it's 0, or higher if it's positive. Adding an extra element, [math]z[/math], just adds a description of where it points in an extra dimension; usually, [math]z[/math] will denote the 3D depth, already given [math]x[/math] and [math]y[/math] for 2D length/height; together they, as [math]\begin{bmatrix}x \\ y \\ z\end{bmatrix}[/math], represent some position in 3 dimensions. For example, [math]\begin{bmatrix}5 \\ 3 \\ 1\end{bmatrix}[/math] will point 5 units (inch, cm, metre, etc) to the right, 3 units up, and 1 unit forward. A matrix [math]\begin{bmatrix}x_{1} & x_{2} & x_{3} \\ y_{1} & y_{2} & y_{3} \\ z_{1} & z_{2} & z_{3}\end{bmatrix}[/math] represents a collection of vectors. Note that you could also represent the matrix as [math]\begin{bmatrix}x_{1} & y_{1} & z_{1} \\ x_{2} & y_{2} & z_{2} \\ x_{3} & y_{3} & z_{3}\end{bmatrix}[/math].
Here's a nice animation illustrating these ideas:
 1
1 -
I disagree with the sentiment that the problem is [math]\frac{x}{0} = y[/math] having multiple solutions (in fact, infinitely many). There are many admissible formulae in our usual algebra that hold several solutions, such as is the case with quadratics; [math]x^{2} = n[/math] will have two for any non-zero [math]n[/math]. This property won't break anything.
The actual problem is that, if division by 0 is given the usual properties of division, then [math]\frac{0}{0}[/math] would equal 1. Still maintaining 0's canonical multiplicative properties, [math]x * 0 = 0[/math]. So, given some non-zero [math]a[/math] and [math]b[/math], [math]a * 0 = b * 0[/math]. Then, as both sides are the same value (= 0), their scaling by some factor [math]n[/math] will yield again the same value on both sides. If [math]n = 0[/math], then we have [math]\frac{a * 0}{0} = \frac{b * 0}{0}[/math], and by our usual rules of multiplication that is equivalent to [math]\frac{0}{0} * a = \frac{0}{0} * b[/math]. Given our admission of 0 to the usual rules/properties as described, we have [math]1 * a = 1 * b[/math], and by multiplicative identity [math]a = b[/math]. That is the problem, I think, or at least a much more salient and convincing one.
0 -
I don't think the matter is the size of the distance, but coordinating the property for more and more runners. It might be trivial for numbers that have trivial properties, such as the smaller ones, but maybe this triviality breaks at eight. Here are some ideas, could it have something to do with 8's being
- The smallest perfect cube greater than 1
- The number of vertices of a cube;
• The number of vertices of a cubical graph, the 3-D case of a hypercube graph - The first composite number that's not a multiple of two primes
- The base of the octonions, the largest generalization of complex numbers (after quaternions)
According to the proof for the case of 7, the problem lies within graph theory, specifically related to graph colouring; maybe the bottleneck lies in the properties related to this.
0 -
It's interesting that one of them (Santilli) has his own Institute for Basic Research, and the other (Corda) runs his International Institute for Theoretical Physics and Mathematics, or IFM. From what I've read, Santilli is fringe, most of his proposals are unverified, but he has developed some technology in application to industrial welding under Magnegas. He also has a bone to pick with one of his colleagues from his Harvard days in the 80's, who apparently shunned Santilli's trying to disprove relativity, and pushed him out. This might be the root of his cranky views.
Corda seems to be reputable in his publications, but I think it's a bit odd that he certifies himself as a full professor at his own institute. There's also a list of proposed scientific advisors on the IFM's website, which actually includes Santilli. In fact, Santilli's institute is listed as a founding organization of the IFM. I'm not familiar with any of the others on the list, but it seems that the IFM isn't affiliated with any particularly reputable academic institution or university, even in Italy. Not that this says anything to the credibility of Corda, but it is often reassuring when someone has the checks and balances of other colleagues in their field, especially if they're on the fringe.
2 -
Well, most languages have the capability to do what you're describing. I think it'd most easily be implemented as a web application that the users can access through their web browsers (IE, Chrome, Firefox, etc). Again, most languages have web development frameworks, so that's not a good criteria either.
Firstly, you'll want to set up a web server, which is like a remote computer that stores the pages of your web site as files, and can be accessed remotely. Like your computer has an IP address, so does the web server, and they're reached by the IP's or domain names like mysite.com or site.net (which really map to the IP addresses). On the server you can install the programming languages and frameworks you'll want to use and write the project there.
I think you'll also want to use a database system, which is like a programming language, but specifically for the purpose of storing and manipulating data. The most popular, and probably good to start with, is SQL, which stores data in tables of columns / rows, like an excel sheet. You can set up the database on your server (by downloading, say, MySQL), and set it commands like
SELECT registration_date FROM users WHERE name="bob46"
To make the front-end, that is what the users see, you'll need to use HTML and CSS, and maybe Javascript. HTML is what you mark up the content of your website with, so as to structure it into types and sections, and CSS is how you style the HTML-structured web page (colours, fonts, sizes, positions). When a page is loaded from a website, the web browser recieves all of the code, reads it, and turns it into text, boxes, shapes, colours, and everything that you see. Javascript lets you do some computation and processing, more than just affecting appearance, directly in the browser, but that shouldn't be your initial concern.
Then, to do the backend, where you handle requests for information and updates to the database, and do calculations that will be returned to the actual page, and everything to that effect, you'll have to use a programming language/web app framework. Here are some possibilities:
PHP - if you want something with a gentle learning curve with which you could build your program quickly. PHP was developed as a web framework exclusively, and though it has tried to shed that shell, it isn't ideal for most other kinds of software.
Python (Flask or Django) - if you want to work with a general purpose language that will likely be applicable to any program you want to make in the future. It is fast, modern (in terms of programming language features), and used from robotics to finance to web development. Flask and Django are its web development frameworks. The learning curve for Python itself is gentle, but fully grasping all of its features and the frameworks will require a tad more effort.
Ruby (Rails) - if you want to work with a very modern and able web framework (Rails), for which the base language (Ruby) can be used as general purpose. Ruby is like Python, but has a steeper learning curve and is a little less powerful in terms of speed and optimization. It received much renewed interest after a revamp (or release, I'm not certain) of the Rails web development framework, which is regarded as top class, providing a better experience in those areas than PHP, Flask, Django, and others.
Node.js - if you want a very modern, straight for the internet, web development framework. It is based on Javascript, the language that is used to make functional front-end, browser driven applications; JS is the standard for doing such things on every widely used web browser today (animations, input/output control, etc). Node.js is essentially an extension of the Javascript empire to the backend, where you could do all of the server-side things the other frameworks can do too.
All of these languages have libraries you can install to communicate with SQL servers, and they generally try to make it easy. At the point where you're familiar with HTML, CSS, SQL, and one of the above languages/frameworks, you could start pumping out web applications of most any kind. Alternatively, you could pick from the above and make a desktop application, rather than a web application, using Python or Rails; in that case you'd have to have each of your users download the program onto their computers.
0 -
...that the "highest" the educational credentials of a person it will be highest the probability of that person rejecting irrationally the reality of anomalies, it is like the "system" had conditioned these people to reject that reality.
What good can be such a system that conditions people to be blind to fundamentally new facts?
The extreme failure of that system is expressed by the continual dismissal of that reality by its highest exponents: Academia and Oficial Science.
Well, it does have some utility; when confronted with uncanny truths, they can wade on with their research unconcerned. You can test this with ease, just message ajb, or swansont, revealing to him the fact of his horrid looks, and he'll move on unperturbed, possibly even outright denying the anomaly! Your post may even be locked up in a secret navy bunker or burried deep in the forests of Poland.
Jokes aside, I think there is a bit, at the elementary and secondary level, of beating curiosity out of kids with tests and standards. They tend to conflate that work with learning and grow to hate it. But often the ones who stay and pursue higher education are those who've seen some value in learning and discover / generate new, unwritten knowledge themselves. Of course, there are others who are more in love with the institution than the study, but they too generally have an appreciation for new results and discoveries.
0 -
I would imagine the regulations would be similar to those for blimps / airships, which are described here: http://www.faa.gov/aircraft/air_cert/design_approvals/airships/airships_regs/.
Also related might be the rules for hot air ballooning, described here: https://www.faa.gov/aircraft/air_cert/design_approvals/balloons/balloons_regs/
There are also regulations for all aircraft within controlled US air space, and a deliniation of what space is "controlled" and what isn't. That refers to air traffic control though, and not national "sovereign space", which a country's laws extend to; as far as I know, there's no set elevation for this.
I don't think we'll have floating cities though; more likely, before the space age, will be artificial islands (seasteads), which would be cheaper than making hovering or underwater cities / homes. Though, we already know what happens when libertarians build sea cities, and patriotic americans build air-villes.
0 -
I don't think that the proletariat of the goods-transportation industry make up a significant portion of the obese population; there are many more who have a daily diet of McDonald's and have less transport intensive jobs.
Automating goods transportation, such as by truck, shouldn't be done, at least for the purpose of avoiding obesity. It will probably happen some day soon, with the advent of self-driving cars, but for efficiency. Taking people's jobs may make them unemployed and hungry, which is much worse, I think, than employed and fat.
0 -
Nice project!
I am not sure I am understanding the failure of the actual mission though. Why did it miss its target?
And, you mentioned that a problem was it couldn't charge itself; how does your system fix this?
0 -
Thanks Sato, that must have taken some time to think of! I wasn't expecting it to get that complacated, although math can get as complex and hard as you want... If it wouldn't take to much time, could you explain to me how you got to the finial answer?
Like how do you know that this will come out with the final answer: If its to tedious and time consuming don't bother, but I am cerious...
How can you explain you came up with the below equation?
[latex] f(x) = ((x \bmod \pi)+\pi) + (x \bmod \pi) * \frac{(\frac{\pi}{2} - (x \bmod \pi)+\pi)}{(x \bmod \pi)^{\lceil \frac{x \bmod \pi}{4}\rceil}} [/latex]Sure,
first, as I said in the formulation, I needed to satisfy the first condition, so found that
[math] (x \bmod \pi)+\pi [/math]
did as needed.
So, that would turn 0 and [math]n\pi[/math] into [math]\pi[/math], which would be evaluated as [math] \sin{\pi} [/math] to be 0.
Then, to satisfy condition two, if it wasn't turned into [math]\pi[/math], it should be turned into [math] \frac{\pi}{2} [/math], which would be evaluated as [math] \sin{\frac{\pi}{2}} [/math] to be 1.
So, given some value a ([math] (x \bmod \pi)+\pi [/math]) that is stuck in an expression, and a value b ([math] \frac{\pi}{2} [/math]) that we want to turn that expression into, what sequence of operations can we use to achieve our goal?
[math]a + b - a[/math]
Or,
[math]((x \bmod \pi)+\pi) + \frac{\pi}{2} - ((x \bmod \pi)+\pi)[/math]
But, unfortunately we find that our first condition is no longer satisfied; it is now always [math]\frac{\pi}{2}[/math], and no longer is it [math]\pi[/math] at 0 and [math]n\pi[/math].
So we want encode that condition as arithmetic, in our expression. That whenever it is 0 or [math]\pi[/math], the replacement of a with b shouldn't happen. We want a value c that can turn off the + b - a. Where a + c(b - a) equals a (that is, c equals 0), whenever we have [math]\pi[/math] or 0. Well, we know that whenever we have one of those values,
[math]( (x \bmod \pi)+\pi) [/math]
evaluates to [math]\pi[/math] as per condition one. So to have it evaluate to 0 when given those values, we'd subtract [math]\pi[/math], which given our expression is not so hard. We then have:
[math] x \bmod \pi [/math]
So that is our c. We then form a + c(b - a), or
[math] ((x \bmod \pi)+\pi) + (x \bmod \pi) * (\frac{\pi}{2} - ((x \bmod \pi)+\pi)) [/math]
This seems good but we find that whenever c doesn't evaluate to 0, it evaluates to the remainder after x (our input) is divided by [math]\pi[/math]. We want c to evaluate to 1 so that c(b - a) is just b - a, but now it's b - a multiplied by some strange number ([math] x \bmod \pi [/math]).
We first consider undoing the effects of c by dividing it out
[math](a + \frac{c(b - a)}{c})[/math]
[math]...[/math]
[math]((x \bmod \pi)+\pi) + (x \bmod \pi) * \frac{(\frac{\pi}{2} - (x \bmod \pi)+\pi)}{(x \bmod \pi)}[/math]
But then it appears that this would make c no longer able in its original use. We want to divide it out for case two where it interferes, but for case one, when c*(b - a) should evaluate to 0, we want c to keep its effect (multiplying by 0). We then notice that 0 cannot be divided out, so case one's (0*(b - a)) / 0 does not have the problem that multiplication by 0 is being undone, but that the expression is being divided by 0, which is undefined and prohibits us from moving on.
We contemplate a solution and become aware our actual goal is to make the denominator non-zero whenever c is 0, and have the denominator be equal to just c whenever c is non-zero (because that means we're in condition two). How can we do this? One idea that comes to mind is that [math]0^0 = 1[/math]. We know that by [math]c = 0[/math], we mean [math](x \bmod \pi) = 0[/math]. So if we raise it to itself,
[math](x \bmod \pi)^{(x \bmod \pi)}[/math]
it will evaluate to 1 ([math]0^0[/math]). However, although this satisfies case one where c will be 0, case two, where c must be divided out, is no longer functioning. Rather, cc is the divisor. This obviously doesn't achieve the same result as dividing by c, as our original "fix" intended. So, we want to raise c in the denominator to some exponent that is equal to c whenever c is 0 (so cc will be 1, avoiding division by 0), and that is equal to 1 whenever c is not 0 (so c1 is just c, as needed for case two).
A wild light bulb appears. We recall the ceiling operation, which takes a decimal number and returns the closest integer greater than it. It comes to mind that if you were to apply the ceiling function to any number greater than 0 and less than or equal to 1, it would always return 1. And if you apply it to an integer it returns that same integer—[math]\lceil 0 \rceil = 0[/math]! So now we have a general way to take a value and return either 0 or 1, so long as it's [math] \geq 0 [/math] and [math] \leq 1 [/math].
We take our specific values and know that some modular operation [math]n \bmod m[/math] cannot evaluate to anything greater than m, so [math] x \bmod \pi [/math] will always be lesser than 3.14159... We also know that given two numbers i and j, if i < j, [math]\frac{i}{j}[/math] will be less than 1. So we can take our c and divide it by some value greater than what c can be, and we will always get a number greater than or equal to 0 and less than 1 (so long as c is non-negative, which it is). We can choose something like 3.15, but 4 is cleaner, so we divide our c by 4. If c is 0, dividing it by 4 will have no effect and will leave it at 0. But, if c > 0, c divided by 4 will yield a decimal greater than 0 but less than one.
Our goal is to make it just c, and so we want to raise our value, whenever it's not 0, to 1. We do this easily by [math]\lceil c \rceil[/math], where whenever it is 0, it will stay 0, and whenever it is a decimal, it will be 1. The expontent comes out as
[math]\lceil \frac{x \bmod \pi}{4}\rceil[/math]
and the final result, putting all of our pieces together, is
[math] ((x \bmod \pi)+\pi) + (x \bmod \pi) * \frac{(\frac{\pi}{2} - (x \bmod \pi)+\pi)}{(x \bmod \pi)^{\lceil \frac{x \bmod \pi}{4}\rceil}} [/math]
I tried to recount my thought process in a very pedagogical way, so there is a good bit of repetition, but hopefully the whole processes as I convey it is comprehensive and comprehensible.
3 -
The piecewise function looks just fine, and cleaner than something more clever or convoluted.
But, if you want to apply sine, you could take your knowledge that
[math]\sin{\frac{\pi}{2}} = 1,\quad \sin{\pi} = 0,\quad \sin{0} = 0,\quad \sin{-\pi} = 0,\quad \sin{-\frac{\pi}{2}} = -1[/math],
and venture to form a function whose restrictions
[math]\{-\pi, 0, \pi\},\quad 0<x\ \land\ x \ne \pi,\quad x<0\ \land\ x \ne -\pi[/math]
respectively have images
[math]\pi,\quad \frac{\pi}{2},\quad -\frac{\pi}{2}[/math]
such as
[math]f(x) = (x \bmod \pi)+\pi[/math]
where the first case is satisfied,
[math]f(x) = ((x \bmod \pi)+\pi) + (x \bmod \pi) * \frac{(\frac{\pi}{2} - (x \bmod \pi)+\pi)}{(x \bmod \pi)^{\lceil \frac{x \bmod \pi}{4}\rceil}}[/math]
where the first and second cases are satisfied,
[math]f(x) = \frac{x}{\left| x \right|^{\lceil \frac{x \bmod \pi}{4}\rceil}}(((x \bmod \pi)+\pi) + (x \bmod \pi) * \frac{(\frac{\pi}{2} - (x \bmod \pi)+\pi)}{(x \bmod \pi)^{\lceil \frac{x \bmod \pi}{4}\rceil}})[/math]
where all three cases are satisfied.
So your sought for function of sine is
[math]f(x) = \sin{\frac{x}{\left| x \right|^{\lceil \frac{x \bmod \pi}{4}\rceil}}(((x \bmod \pi)+\pi) + (x \bmod \pi) * \frac{(\frac{\pi}{2} - (x \bmod \pi)+\pi)}{(x \bmod \pi)^{\lceil \frac{x \bmod \pi}{4}\rceil}})}[/math]
There's your intended function as a function of sine. Note that the mod operator returns the remainder after a division and can be rewritten into an expression involving only the floor function
[math]a \bmod b = a - b * \lfloor \frac{a}{b} \rfloor[/math]
The ceiling function [math]\lceil x \rceil[/math] is like the floor function but it instead chooses the next greatest integer.
I used mod and ceiling for notational convenience, as otherwise the equations would be much more clunky.
Hope this was clear! It was very tedious to come up with, and in the end it is identical to the simple piecewise function. I'd say, stick with simplicity; don't go for the more complicated "clever" solution, if a simpler, more elegant one is available to you.
2



How to become a scientist
in Science Education
Posted
Our thread is getting complicated, i.e. I had to start referring to "snippet #n", and there are several points that are...beside the point. But there is one point you've concluded, that is in direct contradiction to my arguments, and I think should be resolved:
I addressed this in my previous response, maybe you did not see it when writing:
The many technologies that we've found make the learning process, and consequently independent study, easier had only come into being a bit more than a decade ago, and have only become as widespread much more recently. We were only able to dig out a few examples people with no formal training from the last century, none of which I'm sure clipped a "no formal education" badge to their applications and publications. It is now only 15 years into this century, and sooner since the arrival into ubiquity of said technologies. And I've already marked here two examples of "amateur professionals" in their becoming, now in their early 20's who've actually credited specific resources of the internet for much of their circumstance. I'm not sure that I have happened to know every millennial of this sort, and don't think it's so unlikely there are others out there.
I don't expect any more than an extreme minority to fill the journals, but this is because most people who go into science were inclined to it in schooling, and fit sufficiently its pace and methods. Not because nearly everyone of this sort who puts in the sufficient effort fails to be able to make contributions, but because for most people, they fit scholastic bill. And if they don't, it's largely because they're either apathetic to science or aren't able to put in the necessary time and effort to get to that level. But then there is a small minority we have found, epitomized by the likes of Luogeng and Chaitin, who are both interested and able. The reason that the proportion you see is minuscule, is that their proportion to the population of humanity is minuscule. Often a matter of circumstance, not genetics, people who may, may, do better this way should not be discouraged from trying to do so, and this quality should not be so disparaged.