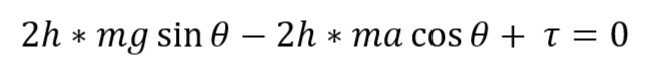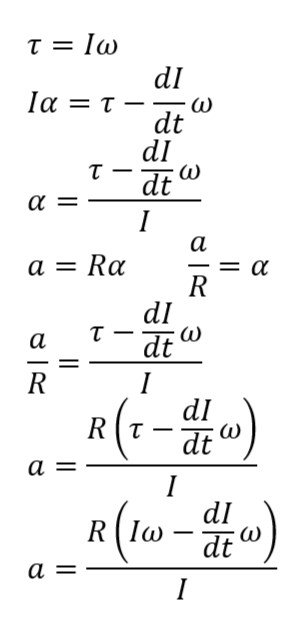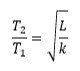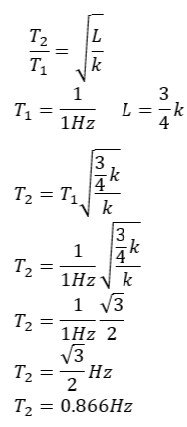Classical Physicist

ok so rolling friction is just F= μR

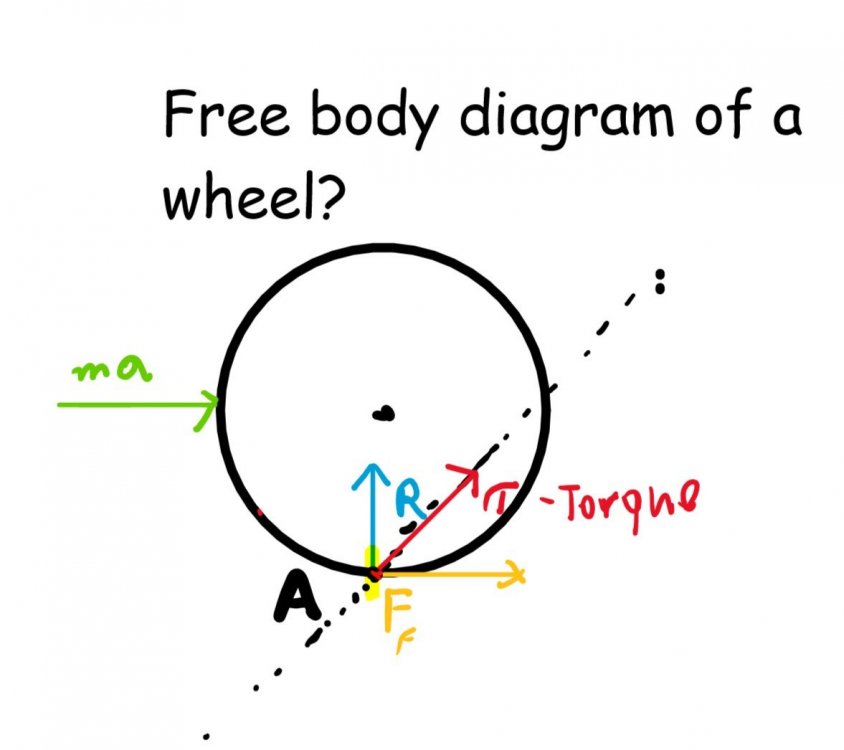
force applied to a wheel F=ma rolling friction is F=-μR normal force R=-mg Is that right? going back to the Two-wheeled inverted pendulum that i have to find acceleration of, by using the point c that is the centre of mass of the whole vehicle, how do I go about finding the torque at that point, which is on a line that is displaced at exactly the center of the rod that is in the axis of rotation of a vehicle… ? I Really need to figure it out @studiot. I can't even tell you how much It would mean to me if we figured it out!

sure But what forces do I need? Torque at the point A, Rolling friction, normal force R, and …. force that is applied to a wheel?

OKAY I need some serious help with it right now. What should I do to find this acceleration?

Yes, It's easier to draw few FBD's for each of the wheels/ points, instead of making complex sketches full of informations… Also what a cute car did You draw! - It's looks just like an Aston Martin! I will send Wheel FBD's here shortly. After this we should go back to finally analyzing the motion, right?

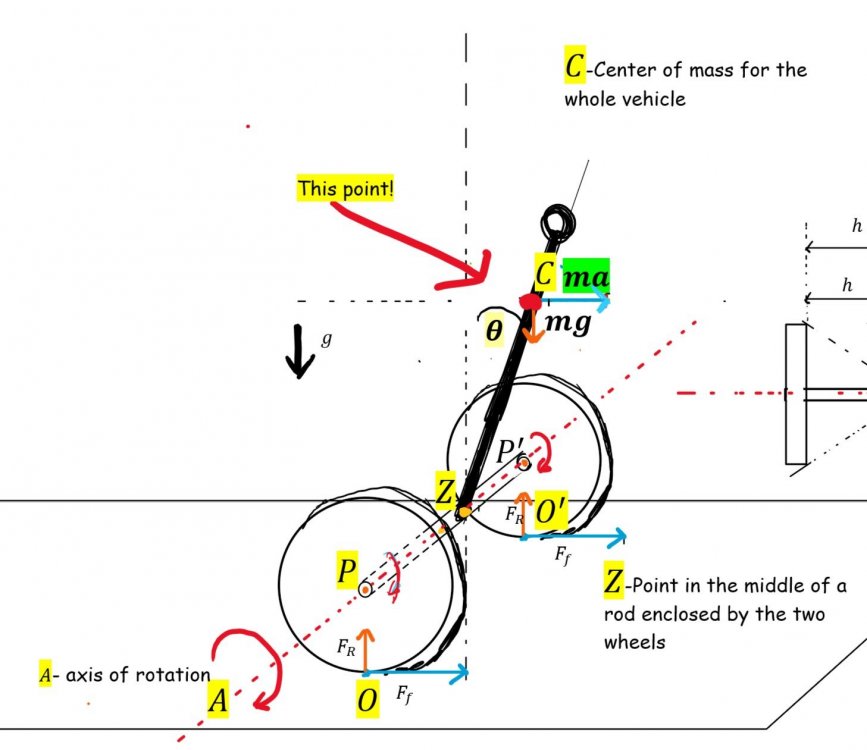
I'd say that the only net forces acting on a point C of a vehicle are torque (2h mg sin θ ) force that gives us acceleration of a vehicle (2h ma cos θ ) and torque of a point C that is τ=Iω Image: Sorry I've been trying to figure it out for days and I still have trouble with applying these forces in order to solve this problem, so I may have a flawed understanding of it right now. As I understand "Rolling motion" is a sum of translational motion and rotational motion. So you suggest starting with finding/composing some equations that will describe the motion of this vehicle? - Sounds correct. I'll see what I can do.

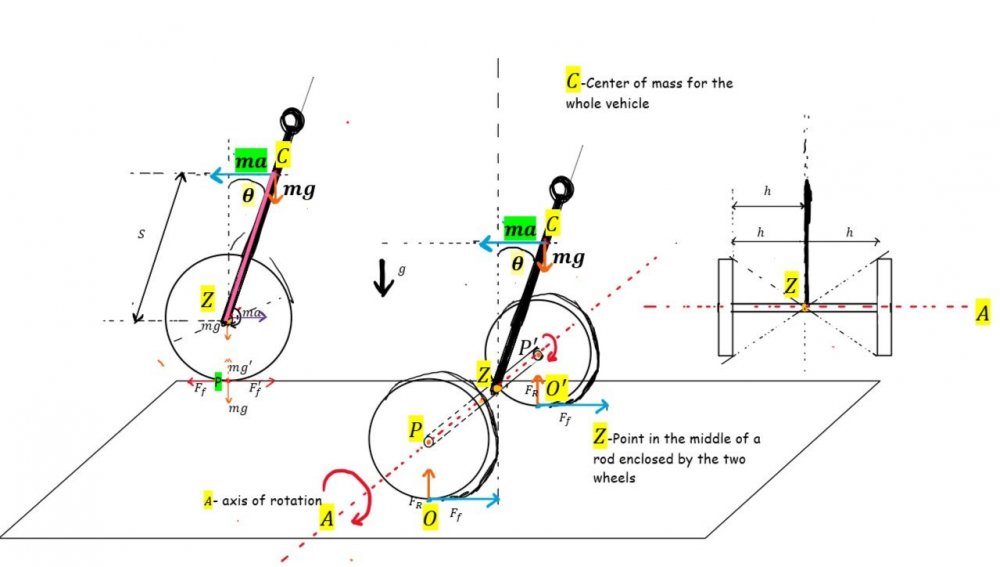
I think I can- but it's really not that simple when there's so many parts here to consider. It simply moves (accelerates uniformly with this unknown yet constant acceleration) in the right direction as the picture suggests. So it's not moving upwards/downwards- just rolling without slipping we assume here that this motion is in the right direction of this horizontal plane. So I think we can say that the equilibrium is met here. I don't see what you tried to post here- is it incomplete? Because you talk here about "the accelerating force on the vehicle" I drew here a non-inertial case of this vehicle in motion - so ma would be the force that it takes to keep the motion going,

Okay so having all of these Drawn out, how can I find acceleration a that is at the point c which is centre of mass of a vehicle like this? @studiot, @Lizwi And am I Missing some important factors here? - θ is the angle that the pink/black rod moves from the equilibrium position that is on the normal line to the surface in order to accelerate a vehicle- something like a segway/ moving inverted pendulum. Thankfully we can omit the rolling resistance - (forces that make the vehicle decelerate) But torque for a point C would have to be a sum of torques acting on this point - right? And from here we would make some equations and transformations in order to find the acceleration a?

Great!, I will look into it in a moment. But first that's what I did to find acceleration of this whole wheel, is it even partially correct? whoops my bad, not sure what happened with formatting of ω either. - reuploaded I'm Reading the chapter You've just sent right now

Let's picture a Rolling without slipping Wheel, that constantly accelerates. radius of a wheel/circle is rr. Basically any wheel of a vehicle is rotating around its fixed axis - AoR -(axis of rotation) Most of the times wheels have this AoR perfectly in the center of the circle/wheel/cylinder. What I want to understand is how can we describe the torque of such a wheel? Because as I understand we could go about finding torque for the center of the circle - point O. I am not sure if it's possible to find the torque for the point P, that is point on a line tangent to the circle on the surface level.

oh I forgot about it so I got this: is this correct?

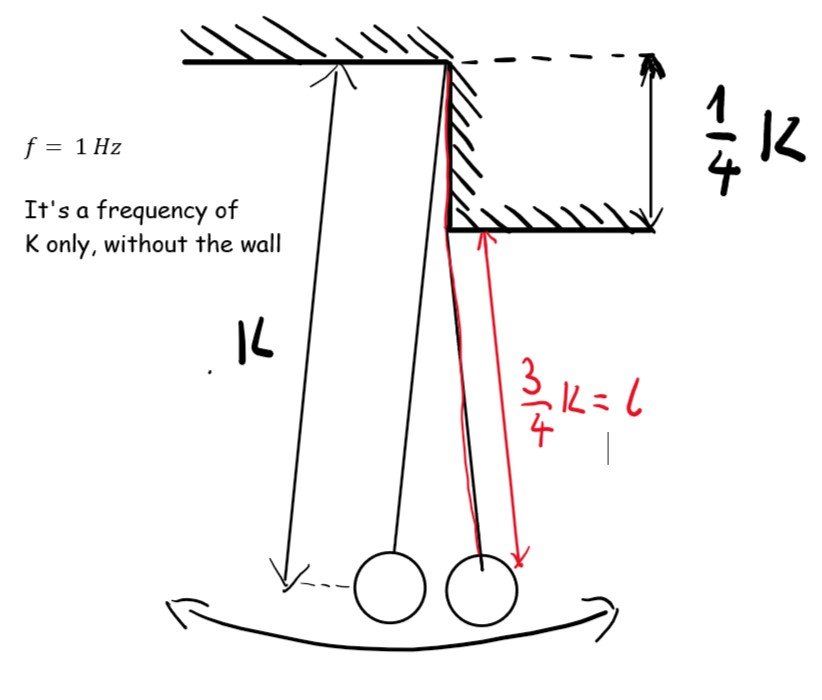
@studiot I guess I have to yet find the third period that is of the overall system so (0.867+1)/2 = T3 That is 0.93?

hey It's me again, I need Help with this pendulum problem, it is easy : The frequency of vibrations (fluctuations) with a small amplitude of the ball attached to a weightless string is 1 Hz. I have to find the frequency of small vibrations of this body suspended on the same string near the edge shown in the picture. The distance d of the edge from the suspension point is 1/4 k. it's easy but i have trouble with comparing it to the "seconds pendulum" Because with seconds pendulum we get frequency f= 1/2 Hz. but here we have so can we compare the two periods like this? so plugging these in I got this: is this correct? @studiot

Love it, Thanks again, I'll post the further answer shortly, …. so how can I insert the maths in here? beause I tried with LateX syntax but it didn't output this nice formatting that you post here?

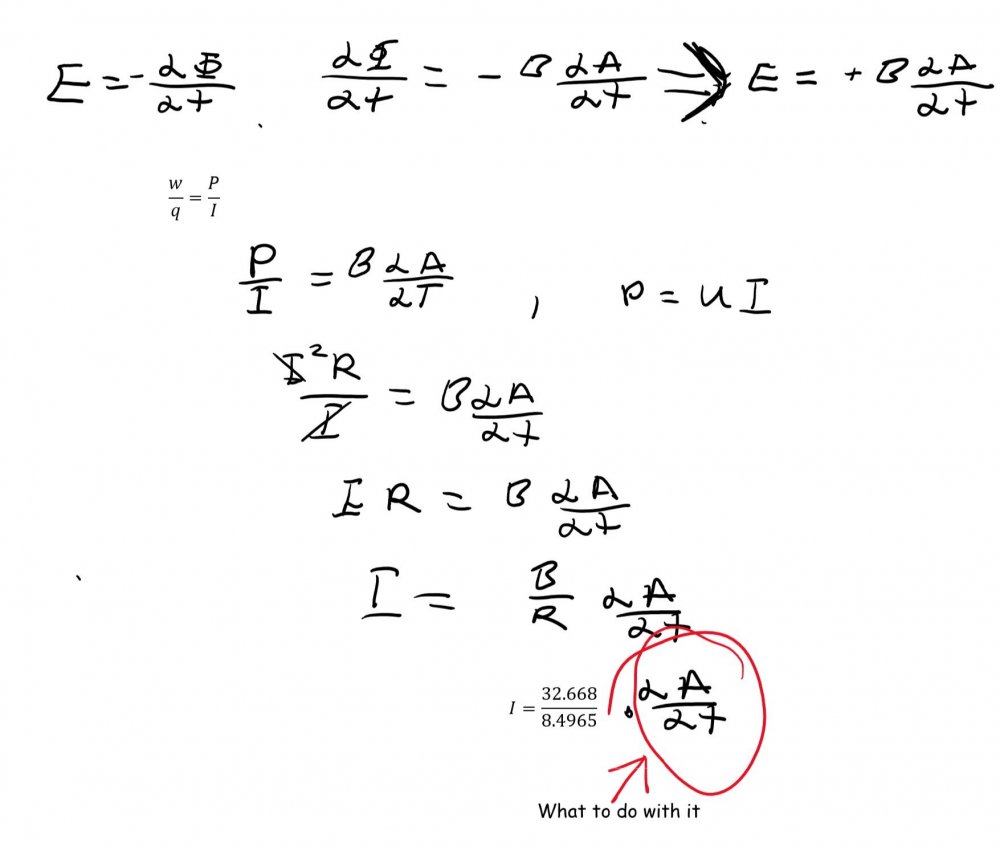
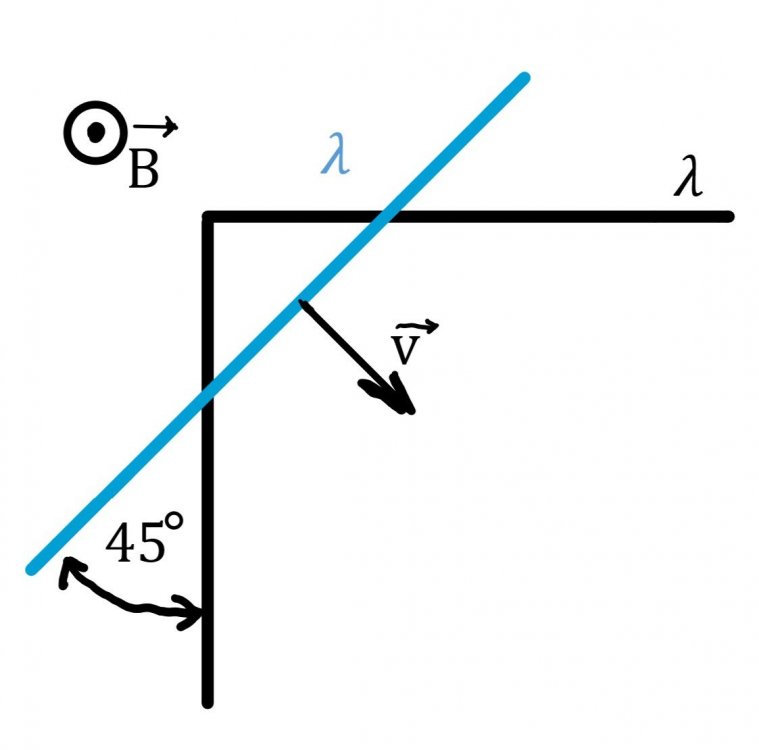
I'm confused The “height” of the triangular area enclosed by the underneath wire and the one above is the same as the distance traveled in time v: d = vt, also the base of the triangle like that equals to λ (as in picture) so we get 1/2* λ*vt=A Do I understand correctly that λ is the length of the wires? - in which case the bent one has λ as a sum of these "sides",so that each side is λ/2? however geometric look at the picture denies that- so the bent part would be 2λ, 1 λ for each side, just like the moving wire above, with l=1 λ. I still don't get which exact triangles should I take into consideration, I mean I understand these ratios, but which triangles with an angle of 45, 45 and 90 from the problem do I need, exactly? Never Thought that I would have to ask you about such trivial stuff, but hey I am very tired right now… However that is still not an excuse.

which chapter is it? the 28th - with magnetic fields, right? Actually the 30th chapter about "Induction and Inductance" is more accurate here, --- you're right the problem 18 from the chapter 30 looks quite similar.

I just got back from Uni and had not much time to sit down to it. But It also boils down to be quite much easier than it appears to be- For a while I was thinking about the whole physics that we could use here, but still your problem solving techniques - about the geometry are still a bit "magic" to me, I get them but, intuitively I would have to practice more to see these just like you do, So the geometry and similarity are well-known to me (or so I suppose), but as you suggested we need to focus on the moving wire that geometrically makes up a triangle with the other wire underneath it. - I sense some differentiation that has to be done with it? we basically have a function of time (?) that with the upper wire movement makes up for an increasing area of a triangle with angles of 45° 45° and 90°. which as time passes by --- reaches the point where the sides are just like in a special triangle with angles like that.

I found it online maybe ...half a year ago, don't really remember the origin of it, it was from some script or notes from/for students of the first year of some course on physics from A university I don't ever so slightly have an idea of (not sure though) that I found while looking for something that could help me understand the electromagnetism better? I don't remember, and the problem was handwritten so yeah, I just got down what you see here.

Yes you're right- that's what it is. two electrical conductors (in a form of wires) with the identical nature.

So I think I need to use the faraday's law here to solve it?

for now I'll have my physics problems posted in "homework help" - because the moderators don't like threads like these in this subtopic

I have a hard time understanding the latter of it- how come the same wire slide upon itself with a velocity, while being inside the magnetic field B? The way I see it- these might not actually be 100% same wire, rather two distinct wires, with the same properties, but being separated? - HOWEVER it wouldn't make sense then, because we need to find the current I, (that is supposedly the same in each "part" of the "wires")… So that is maybe a wrong assumption to begin with. So coming back to the idea of the same wire sliding upon itself - we need to take a look at the basic electromagnetic properties in order to push the problem further? huh, I was going to just do that right now, because I didn't want to clutter the thread, but hey.

A wire with resistance per unit length of 32.668 Ω/m is bent at a right angle. The second straight fragment of the same wire (The blue one) moves at the top of the first one at a speed of v = 4.2735 m/s. The whole system is located in a magnetic field with an induction of B = 8.4965 T, perpendicular to the wires (as in the picture). Determine the current flowing through the wires. Ignore the resistance at the points of contact of both wires and also the magnetic field generated by the current itself that is taken into consideration.

I'm so grateful, for your help, I really get to understand the physics on so much deeper level now as the time goes on, I'll post some more problems in new threads, but as You said -one at a time! Also yeah I Get the significant figures. - so for T=0.40452441211872491886336935537142 s we simply get t=0.405s ,:)

Huge thanks for making a spreadsheet, - so now for sure the sin(45) gives the answer I was looking for. And yeah, I understood the use of double angle formula there, too - a smart way to use it in these type of problems, and I also got the book, and Will certainly have a look, ...explaining calculus problems with some physics sounds very promising! I have some other quite "extraordinary" problems to go through, would you also like to take a look at them?