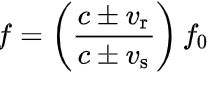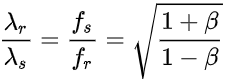All Activity

Utopia is the clue here, sure take steps towards zero but who cares, as long as it's not the final countdown, bc tomorrow we'll be faced with an even bigger stick and it doesn't matter who wields it, good or evil it's all the same in the end... 😉

Travelers dude! Travelers is the show to watch! Time travel is the bomb!

If anything it's the other way round, if for pop-science we say philosophy; science just wants to get on with it, without all those peskie journos asking personal question's, it only gets heated when god is used as an excuse to not try and understand (edit, gods, something else Dawkins was confused by 🙄); if you make an honest mistake the scientists are quite tolerant and happy to explain why. You, my friend is treading a fine line, as did I when I joined this forum and sometimes continue to do so, the difference between now and then is, I learned to listen to these fine people, before I argued with them. I'd hate to see you banned.

Also an advocate for Global Zero here. The Brookings paper makes some strong arguments for continuing to work on this - the mountain seems steeper now, alas, with Putin rekindling the Cold War and saber rattling crazily. I hugely appreciate your passion on this - the world needs to be aware of that Damoclean sword over its head and agitating for its removal. Bingo. Yep. And that's part of why Global Zero is, however distant, a pragmatic approach to global security and species survival. When the stakes are this high, gambling on continued good luck is a bad idea.

Correction applied lol

The 'only time' it was an effective weapon was in Japan; now, it's just a weapon of revenge, whomsoever pulled the trigger first; it's built into the contract, no one can afford to be seen too flinch. If you're facing who you think is a psycho, would you put down your gun? The best we can achieve is for all side's to take out one bullet at a time, the entropy of collateral damage, we'll just be left with one (probably a secret 10) each.

@externo A solid piece of advise. You really need to stop trying to tell us how SR and GR works or describes. We have gone numerous pages with posters correcting your misunderstandings. Which you continue to repeat. I highly suggest that instead of trying to tell us what SR states that instead you start asking questions concerning SR. Use the math and the knowledge of the posters here and try to properly understand SR. This is article was written by a Ph.D that regularly uses forums. He developed this article to provide corrections to all the numerous misconceptions posters regularly have with regards to SR. http://www.lightandmatter.com/sr/ This article describes the basics of SR in a very easy to understand format and explains the reasons behind its mathematics. Relativity: The Special and General Theory" by Albert Einstein http://www.gutenberg.org/files/30155/30155-pdf.pdf It is an archive reprint.

Post what your trying and we can probably help out

Physical time? You keep using expressions like this, and they make no sense. The speed of a wave is frequency*wavelength The frequency increases by the same factor as the wavelength decreases. These terms cancel. The speed of the wave is the same. These are the same thing And you’re wrong. The speed of the wave is constant (it’s right there in the math) since both frequency and wavelength are changed.

(Bold by me) What does 'stationary' mean? Against what? The Lorentzian aether? How can that be, if the LTs do not contain any reference to the velocity of light measured in this 'aether'?

I'm game! Swansont and MigL need to do a deep dive into Farscape lol

https://fr-m-wikipedia-org.translate.goog/wiki/Effet_Doppler?_x_tr_sl=fr&_x_tr_tl=en&_x_tr_hl=fr&_x_tr_pto=wapp#Calcul_relativiste_rapide

It's simple: we synchronize the clocks according to Einstein's procedure so that the one-wat speed of light is measured constant. If this speed is physically constant, the synchronization must indicate the physical time. As each inertial frame has a different synchronization this means that the simultaneity is physically different in each frame. If you think that simultaneity is not physical you also think that the constant one-way speed of light is not physical. So, you see, his speed is not constant relative to the waves... I'm not saying that it doesn't happen as soon as they started moving toward earth, on the contrary, but for it to happen instantly they have to change speed in relation to the waves and therefore the speed of light cannot remain constant when they accelerate, therefore the postulate of the constancy of the speed of light cannot be true. That's all I'm saying. -------------------------------- So you don't have any explanation, you just stick to the math. You used Lorentz mathematics, they are the ones who mathematically resolved the paradox. As for EInstein, to show that he is right you have to prove that the one-way speed of light is constant, which the Dopller effect denies. Why does the frequency of the signal increase as soon as it turns around? Because it is he who causes this increase in the signal by changing its speed relative to the waves. For its part, the Earth must wait for the redshift to propagate at the speed of light to it because its velocity relative to the waves don't change, this is what causes the asymmetry: the speed of light changes relative to the twin which turns around but not relative to the Earth. ---------------------------- The relativistic Doppler effect is calculated assuming that the receiver or transmitter is stationary relative to the medium. In classical physics, if we assume that the receiver is moving, it receives the waves emitted by the source at the frequency: fr = fe (1- v/c) = fe (1- β) with fr = reception frequency and fe emitter frequency If we assume that it is the source which moves, fr = fe/(1+ v/c) = fe/(1+ β) If we add the time dilation factor y² = 1/(1-β²) if we assume that it is the receiver which moves: fr = fe (1- β) * y = sqrt( (1-β)/(1+β)) fe if we assume that it is the transmitter which moves: fr = fe (1- β) / y = sqrt( (1-β)/(1+β)) fe https://fr.wikipedia.org/wiki/Effet_Doppler#Calcul_relativiste_rapide https://fr-m-wikipedia-org.translate.goog/?_x_tr_sl=fr&_x_tr_tl=en&_x_tr_hl=fr&_x_tr_pto=wapp&_x_tr_hist=true#Calcul_relativiste_rapide So the result is identical. If, in classical physics, we assume that the object which moves relative to the medium undergoes time dilation, there is no longer any way to distinguish observationally whether it is the source or the receiver which moves relative to the medium. The relativistic Doppler effect is therefore symmetrical in classical kinematics and we cannot deduce from it that the medium does not exist, but simply that there is observational symmetry and not physical symmetry. And why does the turning twin not need to wait for the signal emitted from Earth to propagate to him before observing the blueshift? He instantly observes it when he turns around because it is he who changes speed in relation to the waves.

Hey @Orion1 welcome back mate. No software I manually type in the latex. Lol thanks for the reminder to keep the metric tensor separate from the Einstein tensor lol

Notice which day this was posted. https://simple.wikipedia.org/wiki/Galactic_year

Thanks. You're right. I also said "produce" when I meant to say "consume".

[math]\color{blue}{\text{Your LaTex Karate has improved, what LaTex software are you using?, just some clarification on formal denotation.}}[/math] [math]\;[/math] [math]\color{blue}{g_{\mu \nu} \text{ and } g_{\alpha \beta} \text{ are formally denoted for the metric spacetime tensor in General Relativity.}}[/math] [math]\color{blue}{G_{\mu \nu} \text{ and } G_{\alpha \beta} \text{ are formally denoted for the Einstein tensor in General Relativity.}}[/math] [math]\;[/math] [math]\color{blue}{\text{The Friedmann–Lemaître–Robertson–Walker FLRW metric:} \; (\text{ref. 1})}[/math] [math]ds^2 = -c \; dt^2 + \frac{a\left(t \right)^2}{1 - k r^2} dr^2 + a\left(t \right)^2 r^2 d \theta^2 + a\left(t \right)^2 r^2 \sin^2 \theta \; d \phi^2[/math] [math]\;[/math] [math]\color{blue}{\text{The metric spacetime tensor in General Relativity for the FLRW metric:} \; (\text{ref. 3, sec. 3.2})}[/math] [math]g_{\mu \nu} = \begin{pmatrix} -1 & 0 & 0 & 0 \\ 0 & \frac{a\left(t \right)^2}{1 - k r^2} & 0 & 0 \\ 0 & 0 & a\left(t \right)^2 r^2 & 0 \\ 0 & 0 & 0 & a\left(t \right)^2 r^2 \sin^2 \theta \end{pmatrix}[/math] [math]\;[/math] [math]\color{blue}{\text{The Einstein tensor in General Relativity:} \; (\text{ref. 2, ref. 3, eq. 3.17})}[/math] [math]G_{\mu \nu } = R_{\mu \nu } - \frac{1}{2} g_{\mu \nu } R[/math] [math]\;[/math] [math]\color{blue}{\text{Any discussions and/or peer reviews about this specific topic thread?}}[/math] [math]\;[/math] Reference: Friedmann-Lemaître-Robertson-Walker metric: (ref. 1) https://en.wikipedia.org/wiki/Friedmann-Lemaître-Robertson-Walker_metric Wikipedia - Einstein tensor (ref. 2) https://en.wikipedia.org/wiki/Einstein_tensor Relativistic Cosmology - M. Pettini: (ref. 3) https://people.ast.cam.ac.uk/~pettini/Intro Cosmology/Lecture03.pdf

I think I found an even better challenge for you, @externo because it seems you have problems with even simple math. This is the formula for the Doppler effect in a medium: vr = is the speed of the receiver relative to the medium, added to ± (above the division) = if the receiver is moving towards the source, subtracted if the receiver is moving away from the source vs = is the speed of the source relative to the medium, added to ± (below the division) if the source is moving away from the receiver, subtracted if the source is moving towards the receiver And last, but not least: c = is the propagation speed of waves in the medium Now this is the Doppler formula for light, assuming the source and the receiver are moving in a straight line from/to each other: where ß is the usual v/c. Now tell me, where do you see the speed of light in a medium? How do you explain that it does not appear in the formula? The above formula, AFAIK, can be derived from the Lorentz transformations, in which, you probably noticed, the speed of light in a medium does not occur either. Another problem I seem to see, is that you are thinking that relativity has something to do with signal delay. It hasn't. So the blueshift that the observer on earth sees after the traveler has turned around, of course takes time to reach earth. That is just signal time delay, nothing special.

Nope, did not work

Except one (or two?) episodes of 'Tales from the Loop' playing with time, it is not the essence of the series, as it is in 'Dark'. The episodes of 'Tales' are relatively independent, but there are a few running threads through the episodes. But maybe this is not the place to discuss that. Maybe the admins could open a new forum for discussing movies and series? Ups, I did not say that!

I never mentioned any U-turn - I made it explicitly clear that I made no assumptions about what the path actually looks like, other than it being light-like (thus differentiable everywhere). Why? Because that’s irrelevant, since the difference in clock readings only depends on the total lengths of the two paths. It’s a global measure along the entire journey. Others here have repeatedly pointed this out too. And since both path length and proper acceleration are invariant measures, both twins agree on the outcome. That’s a meaningless statement. I used Einsteinian SR to show how the twin scenario requires no “resolution”. Really? How do you physically realise an instantaneous U-turn with infinite acceleration? Once again, the clock times are integral measures along the entire journey. In relation to the emitter, not the signal. There’s no rest frame for light.

No, there is no galactic time that we are aware of. I guess I was just postulating on different models for A universe. As it stands it looks like we have one big planet with life on it and the rest stars and other planets. We may find life elsewhere but not yet. The two models mentioned appear to be one in which things develop in to spheres. I can think of a different time where things are triangular centric.

If you start to believe the possible to be impossible, you'll never take steps to achieve what may be achievable. - https://www.brookings.edu/articles/is-a-world-without-nuclear-weapons-really-possible/ That's me all done with this topic for today. Need sleep. Goodnight MigL.

The genie is out of the bottle, and it's too late for wishful thinking. The states that do have such weapons have a history of being provoked to the brink, and haven't used them since 1945. Even new nuclear powers like India, Pakistan and Israel. They are an almost known quantity. It's the unknown quantities like Iran and N Korea that make the equation hard to calculate.

@J.C.MacSwell I apologise if it felt like that was an outburst directed at you. It genuinely wasn't. I'm just frustrated with RL at the moment and not being taken seriously there. It's also late, my back hurts like hell and I've done nothing but mulch raspberry bushes all day. My frustrations are with humanity in general, not anybody here personally and I make these arguments here because this is the only group I trust to follow and understand. I actually didn't. I said drop a nuke on Raffah, which I mispelled, its Rafah and it's the city in southern Gaza the Palestinians are being funneled into. You and me both brother, you and me both. I don't have confidence in any country that has them. I don't want Iran, Israel, Russia, China, the USA or any country to have them. 90 seconds to midnight and not because Iran has nuclear weapons is too close for any comfort or confidence tbh.